Límite de una función
De Wikillerato
| Línea 1: | Línea 1: | ||
| + | %% {{{ =limite | ||
| + | |||
__TOC__ | __TOC__ | ||
| Línea 26: | Línea 28: | ||
| | ||
<math> | <math> | ||
| - | L | + | L \in \mathbb{R} |
</math> | </math> | ||
, es decir | , es decir | ||
| Línea 59: | Línea 61: | ||
tan cercano a | tan cercano a | ||
<math> | <math> | ||
| - | L | + | L \in \mathbb{R} |
</math> | </math> | ||
como queramos eligiendo | como queramos eligiendo | ||
| Línea 91: | Línea 93: | ||
, es | , es | ||
<math> | <math> | ||
| - | L | + | L \in \mathbb{R} |
</math> | </math> | ||
si cualquier sucesión | si cualquier sucesión | ||
| Línea 202: | Línea 204: | ||
[[Category:Matemáticas]] | [[Category:Matemáticas]] | ||
| + | %% }}} | ||
Revisión de 17:20 11 ago 2010
%% {{{ =limite
Tabla de contenidos |
Limite de f(x) cuando x tiende a un número real
El límite de la función
 , cuando
, cuando
 tiende a
tiende a
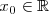 existe y es igual a
existe y es igual a
 , si ambos límites laterales existen y son iguales a
, si ambos límites laterales existen y son iguales a
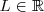 , es decir
, es decir
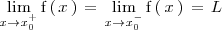
Lo expresamos de la siguiente manera:
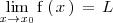
El que la anterior igualdad sea cierta significa que podemos hacer
 tan cercano a
tan cercano a
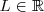 como queramos eligiendo
como queramos eligiendo
 lo suficientemente proximo a
lo suficientemente proximo a
 , por la derecha o por la izquierda.
, por la derecha o por la izquierda.
Limite de f(x) cuando x tiende a infinito
Se dice que el límite de la funcion
 , cuando
, cuando
 tiende a
tiende a
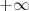 , es
, es
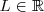 si cualquier sucesión
si cualquier sucesión
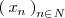 que tiende a
que tiende a
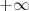 verifica que
verifica que
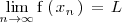 .
.
Lo expresamos como:
[Unparseable or potentially dangerous latex formula. Error 3 ]
El que la anterior igualdad sea cierta significa que podemos hacer
 tan cercano a
tan cercano a
 como queramos eligiendo
como queramos eligiendo
 lo suficientemente grande.
lo suficientemente grande.
Limite de f(x) cuando x tiende a menos infinito
Analogamente, se dice que el límite de la funcion
 , cuando
, cuando
 tiende a
tiende a
 , es
, es
 si cualquier sucesión
si cualquier sucesión
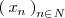 que tiende a
que tiende a
 verifica que
verifica que
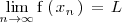 .
.
Lo expresamos como:
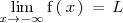
El que la anterior igualdad sea cierta significa que podemos hacer
 tan cercano a
tan cercano a
 como queramos eligiendo
como queramos eligiendo
 lo suficientemente pequeño.
lo suficientemente pequeño.
%% }}}



