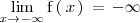Límite de una función
De Wikillerato
| (13 ediciones intermedias no se muestran.) | |||
| Línea 1: | Línea 1: | ||
| - | + | __TOC__ | |
| + | |||
| + | ==Nota sobre terminología== | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Utilizamos la palabra '''''pequeño''''' ( '''''grande''''') de la siguiente manera: | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | <math> | ||
| + | a | ||
| + | </math> | ||
| + | es mas pequeño ( grande ) que | ||
| + | <math> | ||
| + | b | ||
| + | </math> | ||
| + | si y solo si | ||
| + | | ||
| + | <math> | ||
| + | b > a \left( \, a > b \, \right) | ||
| + | </math>. | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Es decir, | ||
| + | <math> | ||
| + | a | ||
| + | </math> | ||
| + | es mas pequeño ( grande ) que | ||
| + | <math> | ||
| + | b | ||
| + | </math> | ||
| + | si | ||
| + | <math> | ||
| + | a | ||
| + | </math> | ||
| + | es menor ( mayor ) que | ||
| + | <math> | ||
| + | b | ||
| + | </math>. | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | La distancia entre dos puntos | ||
| + | <math> | ||
| + | a | ||
| + | </math> | ||
| + | y | ||
| + | <math> | ||
| + | b | ||
| + | </math> | ||
| + | de la recta real | ||
| + | <math> | ||
| + | \left( | ||
| + | \, a, \, b \in \mathbb{R} \, | ||
| + | \right) | ||
| + | </math> | ||
| + | es | ||
| + | <math> | ||
| + | \left| \, a - b \, \right| | ||
| + | </math>. | ||
| + | Cuanto mas pequeña sea esta distancia mas '''''proximos''''' o | ||
| + | '''''cercanos''''' diremos que estan los puntos | ||
| + | <math> | ||
| + | a | ||
| + | </math> | ||
| + | y | ||
| + | <math> | ||
| + | b | ||
| + | </math>. | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Por ejemplo, | ||
| + | <math> | ||
| + | -1 | ||
| + | </math> | ||
| + | esta mas cerca de | ||
| + | <math> | ||
| + | 2 | ||
| + | </math> | ||
| + | que el | ||
| + | <math> | ||
| + | 7 | ||
| + | </math> | ||
| + | ya que | ||
| + | |||
| + | <center> | ||
| + | <math> | ||
| + | \left| \, 2 - 7 \, \right| > \left| \, 2 - \left( -1 \right) \right| | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | ==Limite de f(x) cuando x tiende a un número real== | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | ===Limite finito=== | ||
| + | |||
| + | <br/> | ||
El límite de la función | El límite de la función | ||
| Línea 5: | Línea 107: | ||
\mathrm{f} | \mathrm{f} | ||
</math> | </math> | ||
| - | + | cuando | |
<math> | <math> | ||
x | x | ||
| Línea 11: | Línea 113: | ||
tiende a | tiende a | ||
<math> | <math> | ||
| - | x_0 | + | x_0 \in\mathbb{R} |
</math> | </math> | ||
existe y es igual a | existe y es igual a | ||
<math> | <math> | ||
| - | L | + | L \in \mathbb{R} |
</math> | </math> | ||
| - | + | si ambos [[Límites laterales|límites laterales]] existen y son iguales a | |
| | ||
<math> | <math> | ||
| - | L | + | L |
</math> | </math> | ||
, es decir | , es decir | ||
| Línea 53: | Línea 155: | ||
tan cercano a | tan cercano a | ||
<math> | <math> | ||
| - | L | + | L |
</math> | </math> | ||
como queramos eligiendo | como queramos eligiendo | ||
| Línea 63: | Línea 165: | ||
x_0 | x_0 | ||
</math> | </math> | ||
| - | , por la derecha o por la izquierda. | + | , por la derecha o por la izquierda. |
<br/> | <br/> | ||
| - | + | ===Limite infinito=== | |
<br/> | <br/> | ||
| - | + | El límite de la función | |
<math> | <math> | ||
\mathrm{f} | \mathrm{f} | ||
</math> | </math> | ||
| - | + | cuando | |
<math> | <math> | ||
x | x | ||
| Línea 81: | Línea 183: | ||
tiende a | tiende a | ||
<math> | <math> | ||
| - | + | x_0 \in\mathbb{R} | |
</math> | </math> | ||
| - | + | por la '''''izquierda''''' existe y es igual a | |
<math> | <math> | ||
| - | + | \infty | |
</math> | </math> | ||
| - | + | si podemos hacer | |
<math> | <math> | ||
| - | \left( \, | + | \mathrm{f} \left( \, x \, \right) |
| - | + | ||
</math> | </math> | ||
| - | | + | tan grande como queramos, eligiendo |
<math> | <math> | ||
| - | + | x | |
</math> | </math> | ||
| - | + | lo suficientemente cercano a | |
<math> | <math> | ||
| - | + | x_0 | |
| - | </math>. | + | </math> |
| - | + | por la izquierda | |
| - | + | <math> | |
| - | + | \left( \, x_0 > x \, \right) | |
| - | + | </math> | |
| + | . | ||
<br/> | <br/> | ||
| + | Es decir | ||
<center> | <center> | ||
<math> | <math> | ||
| - | \ | + | \forall y \in \mathbb{R}, \quad \exists \delta > 0 / |
| + | \left( | ||
| + | \, \mathrm{f} \left( \, x \, | ||
| + | \right) > y, \, \quad \forall x \in \left( \, x_0 - \delta, \, x_0 \, \right) | ||
| + | \right) | ||
</math> | </math> | ||
</center> | </center> | ||
| + | |||
| + | Lo expresamos de la siguiente manera: | ||
<br/> | <br/> | ||
| - | + | <center> | |
<math> | <math> | ||
| - | \mathrm{f} \left( \, x \, \right) | + | \lim_{x \to x_0^-} \mathrm{f} \left( \, x \, \right) \, = \, \infty |
</math> | </math> | ||
| - | + | </center> | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | </ | + | |
| - | + | ||
<br/> | <br/> | ||
| - | + | Analogamente, el límite de la función | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | Analogamente, | + | |
<math> | <math> | ||
\mathrm{f} | \mathrm{f} | ||
</math> | </math> | ||
| - | + | cuando | |
<math> | <math> | ||
x | x | ||
| Línea 145: | Línea 242: | ||
tiende a | tiende a | ||
<math> | <math> | ||
| - | + | x_0 \in\mathbb{R} | |
</math> | </math> | ||
| - | + | por la '''''derecha''''' existe y es igual a | |
<math> | <math> | ||
| - | + | \infty | |
</math> | </math> | ||
| - | + | si podemos hacer | |
<math> | <math> | ||
| - | \left( \, | + | \mathrm{f} \left( \, x \, \right) |
| - | + | ||
</math> | </math> | ||
| - | | + | tan grande como queramos, eligiendo |
<math> | <math> | ||
| - | + | x | |
</math> | </math> | ||
| - | + | lo suficientemente cercano a | |
<math> | <math> | ||
| - | + | x_0 | |
| + | </math> | ||
| + | por la derecha | ||
| + | <math> | ||
| + | \left( \, x > x_0 \, \right) | ||
</math>. | </math>. | ||
<br/> | <br/> | ||
| - | Lo expresamos | + | Es decir |
| + | <center> | ||
| + | <math> | ||
| + | \forall y \in \mathbb{R}, \quad \exists \delta > 0 / | ||
| + | \left( | ||
| + | \, \mathrm{f} \left( \, x \, | ||
| + | \right) > y, \, \quad \forall x \in \left( \, x_0, \, x_0 + \delta \, \right) | ||
| + | \right) | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | Lo expresamos de la siguiente manera: | ||
<br/> | <br/> | ||
| Línea 173: | Línea 284: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | \lim_{x \to | + | \lim_{x \to x_0^+} \mathrm{f} \left( \, x \, \right) \, = \, \infty |
</math> | </math> | ||
</center> | </center> | ||
| Línea 179: | Línea 290: | ||
<br/> | <br/> | ||
| - | + | ====Ejemplo==== | |
| + | |||
| + | <br/> | ||
| + | |||
| + | Demostremos que | ||
| + | <center> | ||
<math> | <math> | ||
| - | \ | + | \lim_{x \to 0^+} \frac{1}{x} = \infty |
</math> | </math> | ||
| - | + | </center> | |
| + | |||
| + | Para ello seleccionamos un | ||
<math> | <math> | ||
| - | + | y \in \mathbb{R} | |
</math> | </math> | ||
| - | | + | cualquiera e intentamos encontrar un |
<math> | <math> | ||
| - | + | \delta > 0 | |
</math> | </math> | ||
| - | | + | de manera que |
| - | + | <center> | |
| - | < | + | <math> |
| - | + | x \in \left( \, 0, \, \delta \, \right) \Rightarrow \frac{1}{x} > y | |
| - | + | </math> | |
| - | + | </center> | |
| - | + | ||
| - | + | ||
| - | + | Si | |
<math> | <math> | ||
| - | + | y | |
</math> | </math> | ||
| - | + | no es positivo, entonces cualquier | |
<math> | <math> | ||
| - | + | \delta > 0 | |
</math> | </math> | ||
| - | | + | verifica |
| + | <center> | ||
<math> | <math> | ||
| - | + | x \in \left( \, 0, \, \delta \, \right) \Rightarrow \frac{1}{x} > y | |
| - | \ | + | </math> |
| - | </math> | + | </center> |
| - | + | Si | |
| - | + | ||
| - | + | ||
<math> | <math> | ||
| - | + | y | |
</math> | </math> | ||
| - | + | es positivo, entonces | |
| + | <center> | ||
<math> | <math> | ||
| - | x \ | + | \frac{1}{x} > y \Leftrightarrow \frac{1}{y} > x > 0 |
</math> | </math> | ||
| - | + | </center> | |
| + | |||
| + | Por lo tanto, si elegimos | ||
| + | <center> | ||
<math> | <math> | ||
| - | \ | + | \delta = \frac{1}{y} |
</math> | </math> | ||
| - | + | </center> | |
| + | se verifica que | ||
| + | <center> | ||
<math> | <math> | ||
| - | + | x \in \left( \, 0, \, \delta \, \right) \Rightarrow \frac{1}{x} > y | |
</math> | </math> | ||
| - | + | </center> | |
<br/> | <br/> | ||
| - | + | ===Limite menos infinito=== | |
| - | + | ||
<br/> | <br/> | ||
| - | + | El límite de la función | |
| - | lo | + | <math> |
| + | \mathrm{f} | ||
| + | </math> | ||
| + | cuando | ||
| + | <math> | ||
| + | x | ||
| + | </math> | ||
| + | tiende a | ||
| + | <math> | ||
| + | x_0 \in\mathbb{R} | ||
| + | </math> | ||
| + | por la '''''izquierda''''' existe y es igual a | ||
| + | <math> | ||
| + | -\infty | ||
| + | </math> | ||
| + | si podemos hacer | ||
| + | <math> | ||
| + | \mathrm{f} \left( \, x \, \right) | ||
| + | </math> | ||
| + | tan pequeño como queramos, eligiendo | ||
| + | <math> | ||
| + | x | ||
| + | </math> | ||
| + | lo suficientemente cercano a | ||
| + | <math> | ||
| + | x_0 | ||
| + | </math> | ||
| + | por la izquierda | ||
| + | <math> | ||
| + | \left( \, x_0 > x \, \right) | ||
| + | </math> | ||
| + | . | ||
<br/> | <br/> | ||
| - | + | Es decir | |
| + | <center> | ||
| + | <math> | ||
| + | \forall y \in \mathbb{R}, \quad \exists \delta > 0 / | ||
| + | \left( | ||
| + | \, y > \mathrm{f} \left( \, x \, \right), \, \quad \forall x \in \left( \, x_0 - \delta, \, x_0 \, \right) | ||
| + | \right) | ||
| + | </math> | ||
| + | </center> | ||
| - | + | Lo expresamos de la siguiente manera: | |
| - | + | ||
| - | + | ||
<br/> | <br/> | ||
| - | + | <center> | |
<math> | <math> | ||
| - | x \, = \, | + | \lim_{x \to x_0^-} \mathrm{f} \left( \, x \, \right) \, = \, -\infty |
</math> | </math> | ||
| - | + | </center> | |
| + | |||
| + | <br/> | ||
| + | |||
| + | Analogamente, el límite de la función | ||
<math> | <math> | ||
\mathrm{f} | \mathrm{f} | ||
</math> | </math> | ||
| - | | + | cuando |
| + | <math> | ||
| + | x | ||
| + | </math> | ||
| + | tiende a | ||
| + | <math> | ||
| + | x_0 \in\mathbb{R} | ||
| + | </math> | ||
| + | por la '''''derecha''''' existe y es igual a | ||
| + | <math> | ||
| + | -\infty | ||
| + | </math> | ||
| + | si podemos hacer | ||
| + | <math> | ||
| + | \mathrm{f} \left( \, x \, \right) | ||
| + | </math> | ||
| + | tan pequeño como queramos, eligiendo | ||
| + | <math> | ||
| + | x | ||
| + | </math> | ||
| + | lo suficientemente cercano a | ||
| + | <math> | ||
| + | x_0 | ||
| + | </math> | ||
| + | por la derecha | ||
| + | <math> | ||
| + | \left( | ||
| + | \, x > x_0 \, | ||
| + | \right) | ||
| + | </math>. | ||
<br/> | <br/> | ||
| - | + | Es decir | |
| + | <center> | ||
| + | <math> | ||
| + | \forall y \in \mathbb{R}, \quad \exists \delta > 0 / | ||
| + | \left( | ||
| + | \, \mathrm{f} \left( \, x \, \right) > y, \, \quad \forall x \in \left( \, x_0, \, x_0 + \delta \, \right) | ||
| + | \right) | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | Lo expresamos de la siguiente manera: | ||
<br/> | <br/> | ||
| - | = | + | <center> |
| + | <math> | ||
| + | \lim_{x \to x_0^+} \mathrm{f} \left( \, x \, \right) \, = \, -\infty | ||
| + | </math> | ||
| + | </center> | ||
<br/> | <br/> | ||
| - | + | Cuando alguno de los limites laterales de | |
<math> | <math> | ||
\mathrm{f} | \mathrm{f} | ||
</math> | </math> | ||
| - | + | cuando | |
<math> | <math> | ||
| - | x | + | x |
</math> | </math> | ||
| - | | + | tiende a |
| + | <math> | ||
| + | x_0 | ||
| + | </math> | ||
| + | es infinito o menos infinito, la grafica de | ||
| + | <math> | ||
| + | \mathrm{f} | ||
| + | </math> | ||
| + | tiene una [[Asintotas#Asintotas verticales|asintota vertical]] de ecuación | ||
| + | <math> | ||
| + | x = x_0 | ||
| + | </math>. | ||
<br/> | <br/> | ||
| - | == | + | ==Limite de f(x) cuando x tiende a infinito== |
<br/> | <br/> | ||
| - | + | ===Limite finito=== | |
| + | |||
| + | <br/> | ||
| + | |||
| + | Se dice que el límite de la funcion | ||
<math> | <math> | ||
\mathrm{f} | \mathrm{f} | ||
</math> | </math> | ||
| - | | + | cuando |
| + | <math> | ||
| + | x | ||
| + | </math> | ||
| + | tiende a | ||
| + | <math> | ||
| + | \infty | ||
| + | </math> | ||
| + | es | ||
| + | <math> | ||
| + | L \in \mathbb{R} | ||
| + | </math> | ||
| + | si cualquier sucesión | ||
| + | <math> | ||
| + | \left( \, x_n \, \right) | ||
| + | _{n \in N} | ||
| + | </math> | ||
| + | que tiende a | ||
| + | <math> | ||
| + | \infty | ||
| + | </math> | ||
| + | verifica que | ||
| + | <math> | ||
| + | \lim_{n \to \infty} \mathrm{f} \left( \, x_n \, \right) \, = \, L | ||
| + | </math>. | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Lo expresamos como: | ||
<br/> | <br/> | ||
| Línea 300: | Línea 547: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | \mathrm{f} \left( \, x | + | \lim_{x \to \infty} \mathrm{f} \left( \, x \, \right) \, = \, L |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
</math> | </math> | ||
</center> | </center> | ||
| Línea 314: | Línea 553: | ||
<br/> | <br/> | ||
| - | + | El que la anterior igualdad sea cierta significa que podemos hacer | |
<math> | <math> | ||
| - | + | \mathrm{f} \left( \, x \, \right) | |
</math> | </math> | ||
| - | | + | tan cercano a |
<math> | <math> | ||
| - | + | L | |
</math> | </math> | ||
| - | | + | como queramos eligiendo |
<math> | <math> | ||
| - | + | x | |
</math> | </math> | ||
| - | + | lo suficientemente grande. | |
<br/> | <br/> | ||
| + | Es decir | ||
<center> | <center> | ||
<math> | <math> | ||
| - | \ | + | \forall \epsilon > 0, \quad \exists a \in \mathbb{R} / |
| - | \ | + | \left( |
| + | \, \mathrm{f} \left( \, x \, | ||
| + | \right) \in \left( \, L - \epsilon, \, L + \epsilon \, \right), \, \quad \forall | ||
| + | x \in \left( \, a, \, \infty \, \right) | ||
| + | \right) | ||
</math> | </math> | ||
</center> | </center> | ||
| Línea 339: | Línea 583: | ||
<br/> | <br/> | ||
| - | + | Si el límite de la funcion | |
<math> | <math> | ||
| - | + | \mathrm{f} | |
</math> | </math> | ||
| - | + | cuando | |
<math> | <math> | ||
| - | + | x | |
</math> | </math> | ||
| - | | + | tiende a |
<math> | <math> | ||
| - | + | \infty | |
</math> | </math> | ||
| - | | + | es |
| + | <math> | ||
| + | L \in \mathbb{R} | ||
| + | </math>, | ||
| + | entonces la gráfica de la función | ||
| + | <math> | ||
| + | \mathrm{f} | ||
| + | </math> | ||
| + | tiene una [[Asintotas#Asintotas horizontales|asintota horizontal]] por la | ||
| + | derecha de ecuación | ||
| + | <math> | ||
| + | y = L | ||
| + | </math>. | ||
<br/> | <br/> | ||
| - | === | + | ===Limite infinito=== |
<br/> | <br/> | ||
| - | + | El límite de la función | |
<math> | <math> | ||
| - | + | \mathrm{f} | |
</math> | </math> | ||
| - | + | cuando | |
<math> | <math> | ||
| - | + | x | |
</math> | </math> | ||
| - | | + | tiende a |
<math> | <math> | ||
| - | x \, | + | \infty |
| + | </math> | ||
| + | existe y es igual a | ||
| + | <math> | ||
| + | \infty | ||
| + | </math> | ||
| + | si podemos hacer | ||
| + | <math> | ||
| + | \mathrm{f} \left( \, x \, \right) | ||
| + | </math> | ||
| + | tan grande como queramos, eligiendo | ||
| + | <math> | ||
| + | x | ||
</math> | </math> | ||
| - | + | lo suficientemente grande. | |
<br/> | <br/> | ||
| + | Es decir | ||
<center> | <center> | ||
<math> | <math> | ||
| - | \ | + | \forall y \in \mathbb{R}, \quad \exists a \in \mathbb{R} / |
| - | \ | + | \left( |
| + | \, \mathrm{f} \left( \, x \, | ||
| + | \right) > y, \, \quad \forall x \in \left( \, a, \infty \, \right) | ||
| + | \right) | ||
</math> | </math> | ||
</center> | </center> | ||
| + | |||
| + | Lo expresamos de la siguiente manera: | ||
<br/> | <br/> | ||
| - | = | + | <center> |
| + | <math> | ||
| + | \lim_{x \to \infty} \mathrm{f} \left( \, x \, \right) \, = \, \infty | ||
| + | </math> | ||
| + | </center> | ||
<br/> | <br/> | ||
| - | + | ===Limite menos infinito=== | |
| + | |||
| + | <br/> | ||
| + | |||
| + | El límite de la función | ||
<math> | <math> | ||
\mathrm{f} | \mathrm{f} | ||
</math> | </math> | ||
| - | | + | cuando |
| + | <math> | ||
| + | x | ||
| + | </math> | ||
| + | tiende a | ||
| + | <math> | ||
| + | \infty | ||
| + | </math> | ||
| + | existe y es igual a | ||
| + | <math> | ||
| + | -\infty | ||
| + | </math> | ||
| + | si podemos hacer | ||
| + | <math> | ||
| + | \mathrm{f} \left( \, x \, \right) | ||
| + | </math> | ||
| + | tan pequeño como queramos, eligiendo | ||
| + | <math> | ||
| + | x | ||
| + | </math> | ||
| + | lo suficientemente pequeño. | ||
<br/> | <br/> | ||
| + | Es decir | ||
<center> | <center> | ||
<math> | <math> | ||
| - | \ | + | \forall y \in \mathbb{R}, \quad \exists a \in \mathbb{R} / |
| - | \left | + | \left( |
| - | \ | + | \, y > \mathrm{f} \left( \, x \, |
| - | + | \right), \, \quad \forall x \in \left( \, a, \, \infty \, \right) | |
| - | + | \right) | |
| - | + | ||
| - | + | ||
| - | \right | + | |
</math> | </math> | ||
</center> | </center> | ||
| + | |||
| + | Lo expresamos de la siguiente manera: | ||
<br/> | <br/> | ||
| - | + | <center> | |
| - | < | + | |
| - | + | ||
| - | + | ||
| - | + | ||
<math> | <math> | ||
| - | \lim_{x \to | + | \lim_{x \to -\infty} \mathrm{f} \left( \, x \, \right) \, = \, -\infty |
</math> | </math> | ||
| - | + | </center> | |
<br/> | <br/> | ||
| - | < | + | ==Limite de f(x) cuando x tiende a menos infinito== |
| + | |||
| + | <br/> | ||
| + | |||
| + | ===Limite finito=== | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Se dice que el límite de la funcion | ||
<math> | <math> | ||
| - | + | \mathrm{f} | |
</math> | </math> | ||
| + | cuando | ||
| + | <math> | ||
| + | x | ||
| + | </math> | ||
| + | tiende a | ||
| + | <math> | ||
| + | -\infty | ||
| + | </math> | ||
| + | es | ||
| + | <math> | ||
| + | L \in \mathbb{R} | ||
| + | </math> | ||
| + | si cualquier sucesión | ||
| + | <math> | ||
| + | \left( \, x_n \, \right) | ||
| + | _{n \in N} | ||
| + | </math> | ||
| + | que tiende a | ||
| + | <math> | ||
| + | -\infty | ||
| + | </math> | ||
| + | verifica que | ||
| + | <math> | ||
| + | \lim_{n \to \infty} \mathrm{f} \left( \, x_n \, \right) \, = \, L | ||
| + | </math>. | ||
<br/> | <br/> | ||
| + | Lo expresamos como: | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | <center> | ||
<math> | <math> | ||
| - | \lim_{x \to | + | \lim_{x \to -\infty} \mathrm{f} \left( \, x \, \right) \, = \, L |
</math> | </math> | ||
</center> | </center> | ||
| Línea 437: | Línea 773: | ||
<br/> | <br/> | ||
| - | + | El que la anterior igualdad sea cierta significa que podemos hacer | |
<math> | <math> | ||
| - | \mathrm{f} | + | \mathrm{f} \left( \, x \, \right) |
</math> | </math> | ||
| - | | + | tan cercano a |
<math> | <math> | ||
| - | + | L | |
</math> | </math> | ||
| - | | + | como queramos eligiendo |
| + | <math> | ||
| + | x | ||
| + | </math> | ||
| + | lo suficientemente pequeño. | ||
<br/> | <br/> | ||
| - | + | Es decir | |
| + | <center> | ||
| + | <math> | ||
| + | \forall \epsilon > 0, \quad \exists a \in \mathbb{R} / | ||
| + | \left( | ||
| + | \, \mathrm{f} \left( \, x \, | ||
| + | \right) \in \left( \, L - \epsilon, \, L + \epsilon \, \right), \, \quad \forall x \in \left( \, -\infty, \, a \, \right) | ||
| + | \right) | ||
| + | </math> | ||
| + | </center> | ||
<br/> | <br/> | ||
| - | + | Si el límite de la funcion | |
<math> | <math> | ||
| - | f | + | \mathrm{f} |
</math> | </math> | ||
| - | + | cuando | |
<math> | <math> | ||
| - | x | + | x |
</math> | </math> | ||
| - | | + | tiende a |
<math> | <math> | ||
| - | + | -\infty | |
</math> | </math> | ||
| - | | + | es |
| + | <math> | ||
| + | L \in \mathbb{R} | ||
| + | </math>, | ||
| + | entonces la gráfica de la función | ||
| + | <math> | ||
| + | \mathrm{f} | ||
| + | </math> | ||
| + | tiene una [[Asintotas#Asintotas horizontales|asintota horizontal]] por la | ||
| + | izquierda de ecuación | ||
| + | <math> | ||
| + | y = L | ||
| + | </math>. | ||
<br/> | <br/> | ||
| - | === | + | ===Limite infinito=== |
<br/> | <br/> | ||
| - | + | El límite de la función | |
<math> | <math> | ||
\mathrm{f} | \mathrm{f} | ||
</math> | </math> | ||
| - | | + | cuando |
| + | <math> | ||
| + | x | ||
| + | </math> | ||
| + | tiende a | ||
| + | <math> | ||
| + | -\infty | ||
| + | </math> | ||
| + | existe y es igual a | ||
| + | <math> | ||
| + | \infty | ||
| + | </math> | ||
| + | si podemos hacer | ||
| + | <math> | ||
| + | \mathrm{f} \left( \, x \, \right) | ||
| + | </math> | ||
| + | tan grande como queramos, eligiendo | ||
| + | <math> | ||
| + | x | ||
| + | </math> | ||
| + | lo suficientemente pequeño. | ||
<br/> | <br/> | ||
| + | Es decir | ||
<center> | <center> | ||
<math> | <math> | ||
| - | \ | + | \forall y \in \mathbb{R}, \quad \exists a \in \mathbb{R} / |
| - | \left | + | \left( |
| - | \ | + | \, \mathrm{f} \left( \, x \, |
| - | + | \right) > y, \, \quad \forall x \in \left( \, -\infty, \, a \, \right) | |
| - | + | \right) | |
| - | + | ||
| - | + | ||
| - | \right | + | |
</math> | </math> | ||
</center> | </center> | ||
| + | |||
| + | Lo expresamos de la siguiente manera: | ||
<br/> | <br/> | ||
| - | + | <center> | |
<math> | <math> | ||
| - | x \, = \, | + | \lim_{x \to -\infty} \mathrm{f} \left( \, x \, \right) \, = \, \infty |
</math> | </math> | ||
| - | + | </center> | |
| + | |||
| + | <br/> | ||
| + | |||
| + | ===Limite menos infinito=== | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | El límite de la función | ||
<math> | <math> | ||
| - | + | \mathrm{f} | |
</math> | </math> | ||
| - | + | cuando | |
<math> | <math> | ||
| - | + | x | |
</math> | </math> | ||
| - | | + | tiende a |
<math> | <math> | ||
| - | x \ | + | -\infty |
| - | </math> | + | </math> |
| + | existe y es igual a | ||
| + | <math> | ||
| + | -\infty | ||
| + | </math> | ||
| + | si podemos hacer | ||
| + | <math> | ||
| + | \mathrm{f} \left( \, x \, \right) | ||
| + | </math> | ||
| + | tan pequeño como queramos, eligiendo | ||
| + | <math> | ||
| + | x | ||
| + | </math> | ||
| + | lo suficientemente pequeño. | ||
<br/> | <br/> | ||
| + | Es decir | ||
<center> | <center> | ||
<math> | <math> | ||
| - | \ | + | \forall y \in \mathbb{R}, \quad \exists a \in \mathbb{R} / |
| + | \left( | ||
| + | \, y > \mathrm{f} \left( \, x \, | ||
| + | \right), \, \quad \forall x \in \left( \, -\infty, \, a \, \right) | ||
| + | \right) | ||
</math> | </math> | ||
</center> | </center> | ||
| + | |||
| + | Lo expresamos de la siguiente manera: | ||
<br/> | <br/> | ||
| - | + | <center> | |
<math> | <math> | ||
| - | \mathrm{f} | + | \lim_{x \to -\infty} \mathrm{f} \left( \, x \, \right) \, = \, -\infty |
</math> | </math> | ||
| - | + | </center> | |
| - | + | ||
| - | + | ||
| - | </ | + | |
| - | + | ||
<br/> | <br/> | ||
[[Category:Matemáticas]] | [[Category:Matemáticas]] | ||
Revisión actual
Tabla de contenidos |
Nota sobre terminología
Utilizamos la palabra pequeño ( grande) de la siguiente manera:
 es mas pequeño ( grande ) que
es mas pequeño ( grande ) que
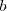 si y solo si
si y solo si
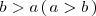 .
.
Es decir,
 es mas pequeño ( grande ) que
es mas pequeño ( grande ) que
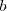 si
si
 es menor ( mayor ) que
es menor ( mayor ) que
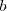 .
.
La distancia entre dos puntos
 y
y
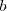 de la recta real
de la recta real
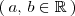 es
es
 .
Cuanto mas pequeña sea esta distancia mas proximos o
cercanos diremos que estan los puntos
.
Cuanto mas pequeña sea esta distancia mas proximos o
cercanos diremos que estan los puntos
 y
y
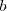 .
.
Por ejemplo,
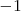 esta mas cerca de
esta mas cerca de
 que el
que el
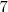 ya que
ya que

Limite de f(x) cuando x tiende a un número real
Limite finito
El límite de la función
 cuando
cuando
 tiende a
tiende a
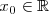 existe y es igual a
existe y es igual a
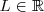 si ambos límites laterales existen y son iguales a
si ambos límites laterales existen y son iguales a
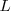 , es decir
, es decir
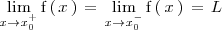
Lo expresamos de la siguiente manera:
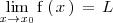
El que la anterior igualdad sea cierta significa que podemos hacer
 tan cercano a
tan cercano a
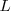 como queramos eligiendo
como queramos eligiendo
 lo suficientemente proximo a
lo suficientemente proximo a
 , por la derecha o por la izquierda.
, por la derecha o por la izquierda.
Limite infinito
El límite de la función
 cuando
cuando
 tiende a
tiende a
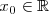 por la izquierda existe y es igual a
por la izquierda existe y es igual a
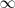 si podemos hacer
si podemos hacer
 tan grande como queramos, eligiendo
tan grande como queramos, eligiendo
 lo suficientemente cercano a
lo suficientemente cercano a
 por la izquierda
por la izquierda
 .
.
Es decir
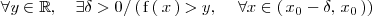
Lo expresamos de la siguiente manera:
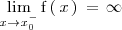
Analogamente, el límite de la función
 cuando
cuando
 tiende a
tiende a
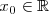 por la derecha existe y es igual a
por la derecha existe y es igual a
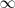 si podemos hacer
si podemos hacer
 tan grande como queramos, eligiendo
tan grande como queramos, eligiendo
 lo suficientemente cercano a
lo suficientemente cercano a
 por la derecha
por la derecha
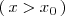 .
.
Es decir
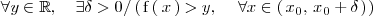
Lo expresamos de la siguiente manera:
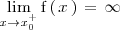
Ejemplo
Demostremos que
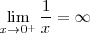
Para ello seleccionamos un
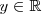 cualquiera e intentamos encontrar un
cualquiera e intentamos encontrar un
 de manera que
de manera que
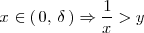
Si
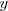 no es positivo, entonces cualquier
no es positivo, entonces cualquier
 verifica
verifica
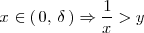
Si
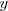 es positivo, entonces
es positivo, entonces
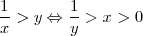
Por lo tanto, si elegimos

se verifica que
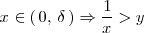
Limite menos infinito
El límite de la función
 cuando
cuando
 tiende a
tiende a
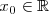 por la izquierda existe y es igual a
por la izquierda existe y es igual a
 si podemos hacer
si podemos hacer
 tan pequeño como queramos, eligiendo
tan pequeño como queramos, eligiendo
 lo suficientemente cercano a
lo suficientemente cercano a
 por la izquierda
por la izquierda
 .
.
Es decir
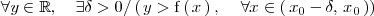
Lo expresamos de la siguiente manera:

Analogamente, el límite de la función
 cuando
cuando
 tiende a
tiende a
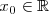 por la derecha existe y es igual a
por la derecha existe y es igual a
 si podemos hacer
si podemos hacer
 tan pequeño como queramos, eligiendo
tan pequeño como queramos, eligiendo
 lo suficientemente cercano a
lo suficientemente cercano a
 por la derecha
por la derecha
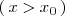 .
.
Es decir
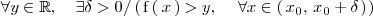
Lo expresamos de la siguiente manera:
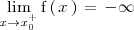
Cuando alguno de los limites laterales de
 cuando
cuando
 tiende a
tiende a
 es infinito o menos infinito, la grafica de
es infinito o menos infinito, la grafica de
 tiene una asintota vertical de ecuación
tiene una asintota vertical de ecuación
 .
.
Limite de f(x) cuando x tiende a infinito
Limite finito
Se dice que el límite de la funcion
 cuando
cuando
 tiende a
tiende a
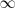 es
es
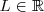 si cualquier sucesión
si cualquier sucesión
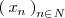 que tiende a
que tiende a
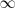 verifica que
verifica que
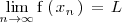 .
.
Lo expresamos como:
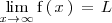
El que la anterior igualdad sea cierta significa que podemos hacer
 tan cercano a
tan cercano a
 como queramos eligiendo
como queramos eligiendo
 lo suficientemente grande.
lo suficientemente grande.
Es decir

Si el límite de la funcion
 cuando
cuando
 tiende a
tiende a
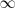 es
es
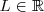 ,
entonces la gráfica de la función
,
entonces la gráfica de la función
 tiene una asintota horizontal por la
derecha de ecuación
tiene una asintota horizontal por la
derecha de ecuación
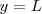 .
.
Limite infinito
El límite de la función
 cuando
cuando
 tiende a
tiende a
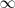 existe y es igual a
existe y es igual a
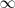 si podemos hacer
si podemos hacer
 tan grande como queramos, eligiendo
tan grande como queramos, eligiendo
 lo suficientemente grande.
lo suficientemente grande.
Es decir
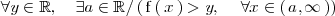
Lo expresamos de la siguiente manera:

Limite menos infinito
El límite de la función
 cuando
cuando
 tiende a
tiende a
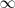 existe y es igual a
existe y es igual a
 si podemos hacer
si podemos hacer
 tan pequeño como queramos, eligiendo
tan pequeño como queramos, eligiendo
 lo suficientemente pequeño.
lo suficientemente pequeño.
Es decir
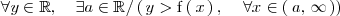
Lo expresamos de la siguiente manera:
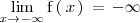
Limite de f(x) cuando x tiende a menos infinito
Limite finito
Se dice que el límite de la funcion
 cuando
cuando
 tiende a
tiende a
 es
es
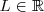 si cualquier sucesión
si cualquier sucesión
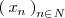 que tiende a
que tiende a
 verifica que
verifica que
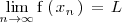 .
.
Lo expresamos como:
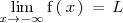
El que la anterior igualdad sea cierta significa que podemos hacer
 tan cercano a
tan cercano a
 como queramos eligiendo
como queramos eligiendo
 lo suficientemente pequeño.
lo suficientemente pequeño.
Es decir
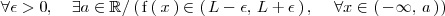
Si el límite de la funcion
 cuando
cuando
 tiende a
tiende a
 es
es
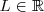 ,
entonces la gráfica de la función
,
entonces la gráfica de la función
 tiene una asintota horizontal por la
izquierda de ecuación
tiene una asintota horizontal por la
izquierda de ecuación
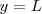 .
.
Limite infinito
El límite de la función
 cuando
cuando
 tiende a
tiende a
 existe y es igual a
existe y es igual a
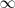 si podemos hacer
si podemos hacer
 tan grande como queramos, eligiendo
tan grande como queramos, eligiendo
 lo suficientemente pequeño.
lo suficientemente pequeño.
Es decir
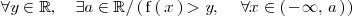
Lo expresamos de la siguiente manera:
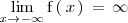
Limite menos infinito
El límite de la función
 cuando
cuando
 tiende a
tiende a
 existe y es igual a
existe y es igual a
 si podemos hacer
si podemos hacer
 tan pequeño como queramos, eligiendo
tan pequeño como queramos, eligiendo
 lo suficientemente pequeño.
lo suficientemente pequeño.
Es decir
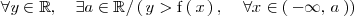
Lo expresamos de la siguiente manera: