Matriz inversa
De Wikillerato
| Línea 1: | Línea 1: | ||
| - | |||
| - | |||
__TOC__ | __TOC__ | ||
| Línea 7: | Línea 5: | ||
<br/> | <br/> | ||
| - | La matriz inversa de una [[ | + | La matriz inversa de una [[¿Qué es una matriz?#Tipos de matrices#Matrices cuadradas|matriz cuadrada]] |
<math> | <math> | ||
\mathbf{A} | \mathbf{A} | ||
| Línea 39: | Línea 37: | ||
\mathbf{I} | \mathbf{I} | ||
</math> | </math> | ||
| - | es la [[ | + | es la |
| + | [[¿Qué es una matriz?#Tipos de matrices#Matrices unidad o identidad|matriz identidad]] de orden | ||
<math> | <math> | ||
n | n | ||
| Línea 51: | Línea 50: | ||
<br/> | <br/> | ||
| - | Las | + | Las matrices que tienen inversa se llaman '''''regulares''''' y las que NO |
tienen inversa, '''''singulares'''''. | tienen inversa, '''''singulares'''''. | ||
| Línea 83: | Línea 82: | ||
<math> | <math> | ||
\left( | \left( | ||
| - | + | \mathbf{A}^{-1} | |
\right) | \right) | ||
^{-1} = \mathbf{A} | ^{-1} = \mathbf{A} | ||
| Línea 93: | Línea 92: | ||
<math> | <math> | ||
\left( | \left( | ||
| - | + | \mathbf{A} \cdot \mathbf{B} | |
\right) | \right) | ||
^{-1} = \mathbf{B}^{-1} \cdot \mathbf{A}^{-1} | ^{-1} = \mathbf{B}^{-1} \cdot \mathbf{A}^{-1} | ||
| Línea 120: | Línea 119: | ||
\mathbf{A} = | \mathbf{A} = | ||
\left( | \left( | ||
| - | + | \begin{array}[c]{cc} | |
| - | + | 1 & 2 | |
| - | + | \\ | |
| - | + | 3 & 7 | |
| - | + | \end{array} | |
\right) | \right) | ||
</math> | </math> | ||
| Línea 139: | Línea 138: | ||
\mathbf{A}^{-1} = | \mathbf{A}^{-1} = | ||
\left( | \left( | ||
| - | + | \begin{array}[c]{cc} | |
| - | + | a & b | |
| - | + | \\ | |
| - | + | c & d | |
| - | + | \end{array} | |
\right) | \right) | ||
</math> | </math> | ||
| Línea 158: | Línea 157: | ||
I = \mathbf{A} \cdot \mathbf{A}^{-1} \Rightarrow | I = \mathbf{A} \cdot \mathbf{A}^{-1} \Rightarrow | ||
\left( | \left( | ||
| - | + | \begin{array}[c]{cc} | |
| - | + | 1 & 2 | |
| - | + | \\ | |
| - | + | 3 & 7 | |
| - | + | \end{array} | |
\right) | \right) | ||
\cdot | \cdot | ||
\left( | \left( | ||
| - | + | \begin{array}[c]{cc} | |
| - | + | a & b | |
| - | + | \\ | |
| - | + | c & d | |
| - | + | \end{array} | |
\right) | \right) | ||
= | = | ||
\left( | \left( | ||
| - | + | \begin{array}[c]{cc} | |
| - | + | 1 & 0 | |
| - | + | \\ | |
| - | + | 0 & 1 | |
| - | + | \end{array} | |
\right) | \right) | ||
</math> | </math> | ||
| Línea 192: | Línea 191: | ||
<math> | <math> | ||
\left( | \left( | ||
| - | + | \begin{array}[c]{cc} | |
| - | + | a + 2c & b + 2d | |
| - | + | \\ | |
| - | + | 3a + 7c & 3b + 7d | |
| - | + | \end{array} | |
\right) | \right) | ||
= | = | ||
\left( | \left( | ||
| - | + | \begin{array}[c]{cc} | |
| - | + | 1 & 0 | |
| - | + | \\ | |
| - | + | 0 & 1 | |
| - | + | \end{array} | |
\right) | \right) | ||
\Leftrightarrow | \Leftrightarrow | ||
\left\{ | \left\{ | ||
| - | + | \begin{array}[c]{ccc} | |
| - | + | a + 2c & = & 1 | |
| - | + | \\ | |
| - | + | 3a + 7c & = & 0 | |
| - | + | \\ | |
| - | + | b + 2d & = & 0 | |
| - | + | \\ | |
| - | + | 3b + 7d & = & 1 | |
| - | + | \\ | |
| - | + | \end{array} | |
\right. | \right. | ||
</math> | </math> | ||
| Línea 227: | Línea 226: | ||
<math> | <math> | ||
\Rightarrow \left\{ | \Rightarrow \left\{ | ||
| - | + | \begin{array}[c]{ccc} | |
| - | + | a & = & 7 | |
| - | + | \\ | |
| - | + | b & = & -2 | |
| - | + | \\ | |
| - | + | c & = & -3 | |
| - | + | \\ | |
| - | + | d & = & 1 | |
| - | + | \\ | |
| - | + | \end{array} | |
\right. | \right. | ||
</math> | </math> | ||
| Línea 243: | Línea 242: | ||
<br/> | <br/> | ||
| - | === | + | ===Por el método de Gauss-Jordan=== |
<br/> | <br/> | ||
| Línea 310: | Línea 309: | ||
s \cdot F_i | s \cdot F_i | ||
</math>. | </math>. | ||
| - | | + | Esta operación la representamos de la |
siguiente forma: | siguiente forma: | ||
Revisión de 08:26 3 oct 2010
Tabla de contenidos |
Definición
La matriz inversa de una matriz cuadrada
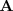 de orden
de orden
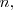 es la matriz cuadrada
es la matriz cuadrada
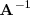 tambien de orden
tambien de orden
 que verifica:
que verifica:
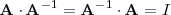
donde
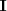 es la
matriz identidad de orden
es la
matriz identidad de orden
 .
.
Exitencia de la matriz inversa
Las matrices que tienen inversa se llaman regulares y las que NO tienen inversa, singulares.
Una matriz cuadrada de orden n es regular si y solo si su rango es n.
Una matriz cuadrada de orden n es singular si y solo si su determinante es cero.
Propiedades
Las propiedades más importantes relativas a la matriz inversa son las siguientes:
1. Si existe,
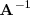 es única.
es única.
2.
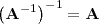
3.
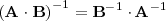
Cálculo de la matriz inversa
La matriz inversa de una matriz regular se puede calcular de diferentes maneras:
Resolviendo un sistema de ecuaciones lineales
Ejemplo
![\mathbf{A} =
\left(
</p>
<pre>\begin{array}[c]{cc}
1 & 2
\\
3 & 7
\end{array}
</pre>
<p>\right)
\mathbf{A} =
\left(
</p>
<pre>\begin{array}[c]{cc}
1 & 2
\\
3 & 7
\end{array}
</pre>
<p>\right)](/images/math/math-d3c460d516d831322ba7600ca96f6a29.png)
hacemos
![\mathbf{A}^{-1} =
\left(
</p>
<pre>\begin{array}[c]{cc}
a & b
\\
c & d
\end{array}
</pre>
<p>\right)
\mathbf{A}^{-1} =
\left(
</p>
<pre>\begin{array}[c]{cc}
a & b
\\
c & d
\end{array}
</pre>
<p>\right)](/images/math/math-b12d162319feb566da12a07314221b42.png)
como
![I = \mathbf{A} \cdot \mathbf{A}^{-1} \Rightarrow
\left(
</p>
<pre>\begin{array}[c]{cc}
1 & 2
\\
3 & 7
\end{array}
</pre>
<p>\right)
\cdot
\left(
</p>
<pre>\begin{array}[c]{cc}
a & b
\\
c & d
\end{array}
</pre>
<p>\right)
=
\left(
</p>
<pre>\begin{array}[c]{cc}
1 & 0
\\
0 & 1
\end{array}
</pre>
<p>\right)
I = \mathbf{A} \cdot \mathbf{A}^{-1} \Rightarrow
\left(
</p>
<pre>\begin{array}[c]{cc}
1 & 2
\\
3 & 7
\end{array}
</pre>
<p>\right)
\cdot
\left(
</p>
<pre>\begin{array}[c]{cc}
a & b
\\
c & d
\end{array}
</pre>
<p>\right)
=
\left(
</p>
<pre>\begin{array}[c]{cc}
1 & 0
\\
0 & 1
\end{array}
</pre>
<p>\right)](/images/math/math-fa501e8cfad8bd98f052c91797b3f2ac.png)
Operando:
![\left(
</p>
<pre>\begin{array}[c]{cc}
a + 2c & b + 2d
\\
3a + 7c & 3b + 7d
\end{array}
</pre>
<p>\right)
=
\left(
</p>
<pre>\begin{array}[c]{cc}
1 & 0
\\
0 & 1
\end{array}
</pre>
<p>\right)
\Leftrightarrow
\left\{
</p>
<pre>\begin{array}[c]{ccc}
a + 2c & = & 1
\\
3a + 7c & = & 0
\\
b + 2d & = & 0
\\
3b + 7d & = & 1
\\
\end{array}
</pre>
<p>\right.
\left(
</p>
<pre>\begin{array}[c]{cc}
a + 2c & b + 2d
\\
3a + 7c & 3b + 7d
\end{array}
</pre>
<p>\right)
=
\left(
</p>
<pre>\begin{array}[c]{cc}
1 & 0
\\
0 & 1
\end{array}
</pre>
<p>\right)
\Leftrightarrow
\left\{
</p>
<pre>\begin{array}[c]{ccc}
a + 2c & = & 1
\\
3a + 7c & = & 0
\\
b + 2d & = & 0
\\
3b + 7d & = & 1
\\
\end{array}
</pre>
<p>\right.](/images/math/math-93a8bef606b968108c4d48ceab054cc5.png)
![\Rightarrow \left\{
</p>
<pre>\begin{array}[c]{ccc}
a & = & 7
\\
b & = & -2
\\
c & = & -3
\\
d & = & 1
\\
\end{array}
</pre>
<p>\right.
\Rightarrow \left\{
</p>
<pre>\begin{array}[c]{ccc}
a & = & 7
\\
b & = & -2
\\
c & = & -3
\\
d & = & 1
\\
\end{array}
</pre>
<p>\right.](/images/math/math-072f3c94488adaa3f7cfe063dd9b30f2.png)
Por el método de Gauss-Jordan
La inversa de una matriz regular
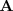 se calcular transformando la matriz
se calcular transformando la matriz
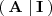 mediante operaciones elementales por filas en la matriz
mediante operaciones elementales por filas en la matriz
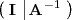
Operaciones elementales por filas en una matriz
Las operaciones elementales por filas en una matriz son las siguientes:
1. Intercambiar las filas
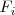 y
y
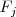 .
Esta operación la representaremos así
.
Esta operación la representaremos así

2. Multiplicar la fila
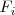 por el número
por el número
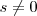 y sustituir
y sustituir
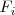 por
por
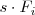 .
Esta operación la representamos de la
siguiente forma:
.
Esta operación la representamos de la
siguiente forma:
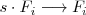
3. Sumar las filas
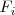 y
y
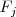 ,
multiplicadas por sendos números,
,
multiplicadas por sendos números,
 y
y
 ,
y sustituir
,
y sustituir
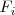 por el resultado de esta suma. Lo representamos así:
por el resultado de esta suma. Lo representamos así:
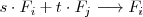
Notese que el segundo tipo de operación,
 ,
es un caso particular de esta última propiedad que se tiene cuando
,
es un caso particular de esta última propiedad que se tiene cuando
 .
.
Mediante la matriz adjunta
Ejercicios resueltos
Producto e invertibilidad de matrices
Tweet


