Métodos de integración
De Wikillerato
| Línea 93: | Línea 93: | ||
</math> | </math> | ||
</center> | </center> | ||
| - | + | se deduce que | |
<center> | <center> | ||
<math> | <math> | ||
| Línea 138: | Línea 138: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | \frac{\mathrm{d}t}{\mathrm{d}x} | + | \mathrm{f}^\prime \left( x \right) = \frac{\mathrm{d}t}{\mathrm{d}x} |
</math> | </math> | ||
</center> | </center> | ||
Ambos miembros de la igualdad anterior son dos formas distintas de denotar la | Ambos miembros de la igualdad anterior son dos formas distintas de denotar la | ||
| - | derivada de la | + | derivada de la función |
<math> | <math> | ||
\mathrm{f} | \mathrm{f} | ||
| Línea 193: | Línea 193: | ||
Supongamos que | Supongamos que | ||
<math> | <math> | ||
| - | \mathrm{G} \left( | + | \mathrm{G} \left( x \right) |
</math> | </math> | ||
es una primitiva de | es una primitiva de | ||
| | ||
<math> | <math> | ||
| - | \mathrm{g} \left( | + | \mathrm{g} \left( x \right) |
</math>, | </math>, | ||
entonces | entonces | ||
| Línea 204: | Línea 204: | ||
<math> | <math> | ||
\int \mathrm{g}^\prime \left( \, \mathrm{f} \left( x \right) \right) \cdot | \int \mathrm{g}^\prime \left( \, \mathrm{f} \left( x \right) \right) \cdot | ||
| - | \mathrm{f}^\prime \left( x \right) | + | \mathrm{f}^\prime \left( x \right) \cdot \mathrm{d}x = \int \mathrm{g} \left( |
| - | t \right) \cdot \mathrm{d}t = \mathrm{G} \left( t \right) + C= \mathrm{ | + | t \right) \cdot \mathrm{d}t = \mathrm{G} \left( t \right) + C= \mathrm{G} \left( |
\mathrm{f} \left( x \right)\right) + C | \mathrm{f} \left( x \right)\right) + C | ||
</math> | </math> | ||
Revisión de 16:19 15 nov 2010
Tabla de contenidos |
Integración por partes
La fórmula para la derivada de un producto es:
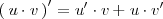
Despejando el último sumando, queda:
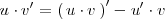
Si integramos en los dos miembros, se obtiene:
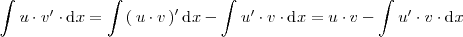
La última igualdad es cierta porque una primitiva de la derivada de una función es esa misma función.
Esta fórmula permite calcular la integral
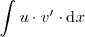 a partir de la integral
a partir de la integral
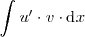 .
.
Para que sea de utilidad el utilizar este metodo es necesario que nos resulte
mas sencilla de resolver la integral
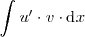 que la integral de partida,
que la integral de partida,
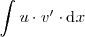 .
.
Ejemplo
Calculemos la integral
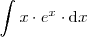
por partes.
Si hacemos
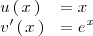
se tiene que
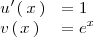
Utilizando la fórmula que hemos visto antes
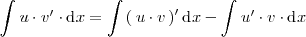
se deduce que
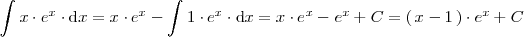
Método de sustitución
Supongamos que queremos resolver una integral del tipo:
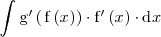
Una manera de resolver un problema de este tipo es haciendo el cambio de variable
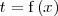
La nueva variable
 es una función de
es una función de
 ,
con lo cual podemos hablar de la derivada de
,
con lo cual podemos hablar de la derivada de
 con respecto de
con respecto de
 , que se puede escribir como un cociente de diferenciales:
, que se puede escribir como un cociente de diferenciales:
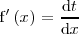
Ambos miembros de la igualdad anterior son dos formas distintas de denotar la
derivada de la función
 .
.
Despejando
 en la igualdad anterior, se deduce que
en la igualdad anterior, se deduce que
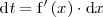
Sustituyendo
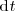 por
por
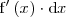 y
y
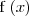 por
por
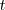 en
en
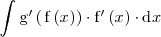
se tiene que
[Unparseable or potentially dangerous latex formula. Error 3 ]
Supongamos que
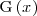 es una primitiva de
es una primitiva de
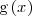 ,
entonces
,
entonces
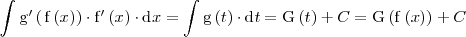
Ejemplo
Calculemos mediante el método de sustitución la integral

Para ello utilizamos las formulas dadas en la descripción del metodo de sustitución con
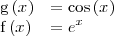
Observese que
[Unparseable or potentially dangerous latex formula. Error 3 ]
En este caso, una primitiva de
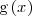 es
es
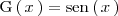
Por lo tanto
[Unparseable or potentially dangerous latex formula. Error 3 ]



