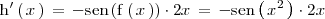Patrocinado por PHPDocX

Síguenos en Twitter

Buscar en WikilleratO
   

Función derivada de la composición de funciones

De Wikillerato

(Diferencias entre revisiones)
Revisión actual (18:48 2 ene 2011) (editar) (deshacer)
 
(6 ediciones intermedias no se muestran.)
Línea 1: Línea 1:
 +
El componer dos funciones  
El componer dos funciones  
<math>
<math>
Línea 9: Línea 10:
&nbsp; consiste en aplicar &nbsp;
&nbsp; consiste en aplicar &nbsp;
<math>
<math>
-
g
+
\mathrm{g}
</math>
</math>
&nbsp; al resultado de calcular &nbsp;
&nbsp; al resultado de calcular &nbsp;
<math>
<math>
\mathrm{f} \left( \, x \, \right)
\mathrm{f} \left( \, x \, \right)
-
</math>
+
</math>:
-
, es decir:
+
<br/>
<br/>
<center>
<center>
-
<math>
 
-
R \stackrel{\mathrm{f}}{\longrightarrow} R \stackrel{\mathrm{g}}{\longrightarrow} R
 
-
</math>
 
-
 
-
<br/>
 
-
 
<math>
<math>
x \longrightarrow \mathrm{f} \left( \, x \, \right) \longrightarrow \mathrm{g}
x \longrightarrow \mathrm{f} \left( \, x \, \right) \longrightarrow \mathrm{g}
\left( \mathrm{f} \left( \, x \, \right) \right)
\left( \mathrm{f} \left( \, x \, \right) \right)
</math>
</math>
-
 
</center>
</center>
Línea 45: Línea 38:
<center>
<center>
<math>
<math>
-
\mathrm{g}^\prime \left( \mathrm{f} \left( \, x \, \right) \right) \cdot \mathrm{f}^\prime \left( \, x \, \right)
+
\left(
 +
\, \mathrm{g} \left( \mathrm{f} \left( \, x \, \right) \right)
 +
\right)
 +
^\prime \, = \, \mathrm{g}^\prime \left( \mathrm{f} \left( \, x \, \right) \right) \cdot \mathrm{f}^\prime \left( \, x \, \right)
</math>
</math>
</center>
</center>
Línea 52: Línea 48:
resultado que se conoce como '''''regla de la cadena'''''.
resultado que se conoce como '''''regla de la cadena'''''.
 +
 +
<br/>
 +
 +
==Ejemplo==
 +
 +
<br/>
 +
 +
Calculemos la derivada de
 +
 +
<br/>
 +
 +
<center>
 +
<math>
 +
\mathrm{h} \left( \, x \, \right) \, = \, \cos \left( \, x^2 \, \right)
 +
</math>
 +
</center>
 +
 +
<br/>
 +
 +
&nbsp;
 +
<math>
 +
\mathrm{h}
 +
</math>
 +
&nbsp; es la composición de dos funciones:
 +
 +
<br/>
 +
 +
<center>
 +
<math>
 +
\left\{
 +
\begin{array}[c]{rcl}
 +
\mathrm{f} \left( \, x \, \right) \, & = & \, x^2
 +
\\
 +
\mathrm{g} \left( \, x \, \right) \, & = & \, \cos \left( \, x \, \right)
 +
\end{array}
 +
\right.
 +
</math>
 +
</center>
 +
 +
<br/>
 +
 +
Es decir
 +
 +
<br/>
 +
 +
<center>
 +
<math>
 +
\mathrm{h} \left( \, x \, \right) \, = \, \mathrm{g} \left( \, \mathrm{f} \left( \, x \, \right) \right)
 +
</math>
 +
</center>
 +
 +
<br/>
 +
 +
Para derivar &nbsp;
 +
<math>
 +
\mathrm{h} \left( \, x \, \right)
 +
</math>
 +
&nbsp; utilizamos la regla de la cadena:
 +
 +
<br/>
 +
 +
<center>
 +
<math>
 +
\mathrm{h}^\prime \left( \, x \, \right) \, = \, \mathrm{g}^\prime \left( \, \mathrm{f} \left( \, x \, \right) \right) \cdot \mathrm{f}^\prime \left( \, x \, \right)
 +
</math>
 +
</center>
 +
 +
<br/>
 +
 +
Como
 +
 +
<br/>
 +
 +
<center>
 +
<math>
 +
\left\{
 +
\begin{array}[c]{rcl}
 +
\mathrm{f}^\prime \left( \, x \, \right) \, & = & \, 2x
 +
\\
 +
\mathrm{g}^\prime \left( \, x \, \right) \, & = & \, -\mathrm{sen} \left( \, x \, \right)
 +
\end{array}
 +
\right.
 +
</math>
 +
</center>
 +
 +
<br/>
 +
 +
se tiene que
 +
 +
<br/>
 +
 +
<center>
 +
<math>
 +
\mathrm{h}^\prime \left( \, x \, \right) \, = \, -\mathrm{sen} \left(
 +
\mathrm{f} \left( \, x \, \right) \right) \cdot 2x \, = \, -\mathrm{sen} \left(
 +
\, x^2 \, \right) \cdot 2x
 +
</math>
 +
</center>
<br/>
<br/>
[[Category:Matemáticas]]
[[Category:Matemáticas]]

Revisión actual

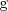El componer dos funciones   \mathrm{f}   y   \mathrm{g}   consiste en aplicar 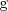  \mathrm{g}   al resultado de calcular   \mathrm{f} \left( \, x \, \right) :


x \longrightarrow \mathrm{f} \left( \, x \, \right) \longrightarrow \mathrm{g} \left( \mathrm{f} \left( \, x \, \right) \right)


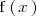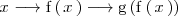
La derivada de 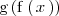  \mathrm{g} \left( \mathrm{f} \left( \, x \, \right) \right)   viene dada por la fórmula: 



\left(
   \, \mathrm{g} \left( \mathrm{f} \left( \, x \, \right) \right)
\right)
^\prime \, = \, \mathrm{g}^\prime \left( \mathrm{f} \left( \, x \, \right) \right) \cdot \mathrm{f}^\prime \left( \, x \, \right)


resultado que se conoce como regla de la cadena.


Ejemplo


Calculemos la derivada de


\mathrm{h} \left( \, x \, \right) \, = \, \cos \left( \, x^2 \, \right)


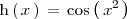
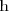  \mathrm{h}   es la composición de dos funciones:


\left\{ </p> <pre> \begin{array}[c]{rcl} \mathrm{f} \left( \, x \, \right) \, & = & \, x^2 \\ \mathrm{g} \left( \, x \, \right) \, & = & \, \cos \left( \, x \, \right) \end{array} </pre> <p>\right.


Es decir


\mathrm{h} \left( \, x \, \right) \, = \, \mathrm{g} \left( \, \mathrm{f} \left( \, x \, \right) \right)


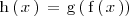
Para derivar 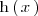  \mathrm{h} \left( \, x \, \right)   utilizamos la regla de la cadena:


</p> <pre> \mathrm{h}^\prime \left( \, x \, \right) \, = \, \mathrm{g}^\prime \left( \, \mathrm{f} \left( \, x \, \right) \right) \cdot \mathrm{f}^\prime \left( \, x \, \right) </pre> <p>


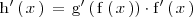
Como


\left\{ </p> <pre> \begin{array}[c]{rcl} \mathrm{f}^\prime \left( \, x \, \right) \, & = & \, 2x \\ \mathrm{g}^\prime \left( \, x \, \right) \, & = & \, -\mathrm{sen} \left( \, x \, \right) \end{array} </pre> <p>\right.


se tiene que


\mathrm{h}^\prime \left( \, x \, \right) \, = \, -\mathrm{sen} \left( </p> <pre> \mathrm{f} \left( \, x \, \right) \right) \cdot 2x \, = \, -\mathrm{sen} \left( \, x^2 \, \right) \cdot 2x </pre> <p>


Obtenido de "http://wikillerato.org/Funci%C3%B3n_derivada_de_la_composici%C3%B3n_de_funciones.html"
   
 
ASIGNATURAS
 MatemáticasFísicaQuímicaBiologíaDibujoHistoriaLengua y LiteraturaHistoria del ArteFilosofía
Creative Commons License
Los contenidos de Wikillerato están disponibles bajo una licencia de Creative Commons.
Pueden utilizarse y redistribuirse libremente siempre que se reconozca su procedencia.