Continuidad de una función
De Wikillerato
(Diferencias entre revisiones)
(→Ejercicios Resueltos) |
(→Ejercicios resueltos) |
||
| (4 ediciones intermedias no se muestran.) | |||
| Línea 1: | Línea 1: | ||
| - | Una función | + | __TOC__ |
| + | |||
| + | ==Definiciones== | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Una función <math> | ||
| + | \mathrm{f} | ||
| + | </math> es '''''continua''''' en <math> | ||
| + | x \, = \, x_0 | ||
| + | </math> si y solo si <math> | ||
| + | \lim_{x \to x_0} \mathrm{f} \left( \, x \, \right) \, = \, | ||
| + | \mathrm{f} \left( \, x_0 \, \right) | ||
| + | </math>. | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Una función | ||
<math> | <math> | ||
\mathrm{f} | \mathrm{f} | ||
</math> | </math> | ||
| - | + | es '''''continua en un intervalo''''' | |
<math> | <math> | ||
| - | + | \left( | |
| + | \, a, \, b \, | ||
| + | \right) | ||
</math> | </math> | ||
| - | si | + | si y solo si |
<math> | <math> | ||
| - | + | \mathrm{f} | |
| - | \ | + | </math> |
| + | es continua en | ||
| + | <math> | ||
| + | x, \, \forall x \in \left( \, a, \, b \, \right) | ||
</math>. | </math>. | ||
<br/> | <br/> | ||
| - | |||
<math> | <math> | ||
| - | \ | + | \forall |
</math> | </math> | ||
| - | + | es un simbolo matematico que significa '''''para todo'''''. | |
| + | |||
| + | <br/> | ||
| + | |||
| + | Se dice que una función | ||
<math> | <math> | ||
| - | + | \mathrm{f} \left( \, x \, \right) | |
</math> | </math> | ||
| - | | + | es '''''continua''''' si y solo si es continua en cada |
<math> | <math> | ||
| - | + | x | |
</math> | </math> | ||
| - | + | de su dominio. | |
| + | |||
| + | <br/> | ||
| + | |||
| + | ==¿Donde es f continua?== | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Para determinar si una función | ||
<math> | <math> | ||
| - | + | \mathrm{f} | |
| + | </math> | ||
| + | es continua en | ||
| + | <math> | ||
| + | x = x_0 | ||
</math> | </math> | ||
| - | | + | comprobaremos que se verifican todas y cada una de las condiciones siguientes: |
<br/> | <br/> | ||
| - | + | ====Condición 1.==== | |
| - | + | ||
| + | Existe <math> | ||
| + | \mathrm{f} \left( \, x_0 \, \right) | ||
| + | </math>, | ||
| + | es decir, <math> | ||
| + | x_0 | ||
| + | </math> | ||
| + | esta en el dominio de <math> | ||
| + | \mathrm{f} | ||
| + | </math>. | ||
<br/> | <br/> | ||
| - | + | ====Condición 2.==== | |
| - | + | ||
| + | Existe <math> | ||
| + | \lim_{x \to x_0} \mathrm{f} \left( \, x \, \right) | ||
| + | </math>. | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | ====Condición 3.==== | ||
| + | |||
| + | Ambos, <math> | ||
| + | \mathrm{f} \left( \, x_0 \, \right) | ||
| + | </math> y <math> | ||
| + | \lim_{x \to x_0} \mathrm{f} \left( \, x \, \right) | ||
| + | </math> | ||
| + | | ||
| + | son iguales. | ||
<br/> | <br/> | ||
| - | ==Ejercicios | + | ==Ejercicios resueltos== |
| - | + | ||
| - | [http://www. | + | {{problemas}}* [http://www.selectividad.tv/S_M_2_1_16_S_continuidad_y_derivabilidad_de_una_funcion.html Continuidad y derivabilidad de una función] |
| - | + | * [http://www.selectividad.tv/S_M_2_1_2_S_continuidad_y_derivabilidad_de_una_funcion_definida_a_trozos.html Continuidad y derivabilidad de una función definida a trozos] | |
| - | [http://www. | + | |
| - | + | ||
[[Category:Matemáticas]] | [[Category:Matemáticas]] | ||
Revisión actual
Tabla de contenidos |
Definiciones
Una funciónes continua en
si y solo si
.
Una función
 es continua en un intervalo
es continua en un intervalo
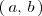 si y solo si
si y solo si
 es continua en
es continua en
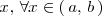 .
.
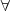 es un simbolo matematico que significa para todo.
es un simbolo matematico que significa para todo.
Se dice que una función
 es continua si y solo si es continua en cada
es continua si y solo si es continua en cada
 de su dominio.
de su dominio.
¿Donde es f continua?
Para determinar si una función
 es continua en
es continua en
 comprobaremos que se verifican todas y cada una de las condiciones siguientes:
comprobaremos que se verifican todas y cada una de las condiciones siguientes:
Condición 1.
Existe 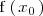 ,
es decir,
,
es decir,  esta en el dominio de
esta en el dominio de  .
.
Condición 2.
Existe 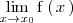 .
.
Condición 3.
Ambos, 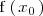 y
y 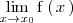 son iguales.
son iguales.
Ejercicios resueltos
También te pueden interesar los siguientes ejercicios resueltos de selectividad
- Continuidad y derivabilidad de una función
- Continuidad y derivabilidad de una función definida a trozos



