Conceptos básicos: espacios vectoriales
De Wikillerato
m (Revertidas las ediciones realizadas por 190.206.25.54 (Talk); a la última edición de 80.58.205.40) |
|||
| (24 ediciones intermedias no se muestran.) | |||
| Línea 1: | Línea 1: | ||
| + | ==Vectores en el plano== | ||
| + | |||
| + | <br/> | ||
| + | |||
En el plano, un vector fijo | En el plano, un vector fijo | ||
<math> | <math> | ||
| Línea 15: | Línea 19: | ||
<br/> | <br/> | ||
| - | < | + | <span |
| - | + | style = 'color:#00aa00'> | |
| - | </ | + | • |
| + | </span> | ||
Módulo: longitud del segmento | Módulo: longitud del segmento | ||
<math> | <math> | ||
| Línea 25: | Línea 30: | ||
<br/> | <br/> | ||
| - | < | + | <span |
| - | + | style = 'color:#00aa00'> | |
| - | </ | + | • |
| + | </span> | ||
Dirección: la de la recta que lo contiene y todas sus paralelas. | Dirección: la de la recta que lo contiene y todas sus paralelas. | ||
<br/> | <br/> | ||
| - | < | + | <span |
| - | + | style = 'color:#00aa00'> | |
| - | </ | + | • |
| + | </span> | ||
Sentido: el que va del origen al extremo. | Sentido: el que va del origen al extremo. | ||
| Línea 107: | Línea 114: | ||
</math> | </math> | ||
del plano en un sistema de coordenadas cartesiano | del plano en un sistema de coordenadas cartesiano | ||
| - | se trazan | + | se trazan desde |
<math> | <math> | ||
P | P | ||
| Línea 157: | Línea 164: | ||
. Se escribe | . Se escribe | ||
<math> | <math> | ||
| - | P = | + | P \, = \, |
\left( | \left( | ||
\, x_1, \, y_1 \, | \, x_1, \, y_1 \, | ||
| Línea 176: | Línea 183: | ||
Conocidas las coordenadas del origen | Conocidas las coordenadas del origen | ||
<math> | <math> | ||
| - | A = | + | A \, = \, |
\left( | \left( | ||
\, x_1, \, y_1 \, | \, x_1, \, y_1 \, | ||
| Línea 183: | Línea 190: | ||
y del extremo | y del extremo | ||
<math> | <math> | ||
| - | B = | + | B \, = \, |
\left( | \left( | ||
\, x_2, \, y_2 \, | \, x_2, \, y_2 \, | ||
| Línea 199: | Línea 206: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | \stackrel{\longrightarrow}{AB} = | + | \stackrel{\longrightarrow}{AB} \, = \, |
\left( | \left( | ||
| - | \, x_2 - x_1, \, y_2 - y_1 \, | + | \, x_2 \, - \, x_1, \, y_2 \, - \, y_1 \, |
\right) | \right) | ||
</math> | </math> | ||
| Línea 222: | Línea 229: | ||
dos vectores libres, se define el vector suma | dos vectores libres, se define el vector suma | ||
<math> | <math> | ||
| - | \vec{\mathbf{u}} + \vec{\mathbf{v}} | + | \vec{\mathbf{u}} \, + \, \vec{\mathbf{v}} |
</math> | </math> | ||
como otro vector obtenido de la siguiente forma: | como otro vector obtenido de la siguiente forma: | ||
| Línea 228: | Línea 235: | ||
<br/> | <br/> | ||
| - | 1. Se señala un punto | + | |
| + | <span | ||
| + | style = 'color:#00aa00'> | ||
| + | 1. | ||
| + | </span> | ||
| + | Se señala un punto | ||
<math> | <math> | ||
O | O | ||
| Línea 244: | Línea 256: | ||
<br/> | <br/> | ||
| - | 2. Por el extremo | + | <span |
| + | style = 'color:#00aa00'> | ||
| + | 2. | ||
| + | </span> | ||
| + | Por el extremo | ||
<math> | <math> | ||
P | P | ||
| Línea 255: | Línea 271: | ||
<br/> | <br/> | ||
| - | 3. El vector | + | <span |
| + | style = 'color:#00aa00'> | ||
| + | 3. | ||
| + | </span> | ||
| + | El vector | ||
<math> | <math> | ||
\stackrel{\longrightarrow}{OQ} | \stackrel{\longrightarrow}{OQ} | ||
| Línea 269: | Línea 289: | ||
( extremo del segundo ) es el representante del vector suma | ( extremo del segundo ) es el representante del vector suma | ||
<math> | <math> | ||
| - | \vec{u} + \vec{v} | + | \vec{u} \, + \, \vec{v} |
</math> | </math> | ||
. | . | ||
| Línea 285: | Línea 305: | ||
<br/> | <br/> | ||
| - | < | + | <span |
| - | + | style = 'color:#00aa00'> | |
| - | </ | + | • |
| - | | + | </span> |
| + | Asociativa: | ||
<math> | <math> | ||
\left( | \left( | ||
| - | \, \vec{\mathbf{u}} + \vec{\mathbf{v}} \, | + | \, \vec{\mathbf{u}} \, + \, \vec{\mathbf{v}} \, |
\right) | \right) | ||
| - | + \vec{\mathbf{w}} = | + | \, + \, \vec{\mathbf{w}} \, = \, |
| - | \vec{\mathbf{u}} + | + | \vec{\mathbf{u}} \, + \, |
\left( | \left( | ||
| - | \, \vec{\mathbf{v}} + \vec{\mathbf{w}} \, | + | \, \vec{\mathbf{v}} \, + \, \vec{\mathbf{w}} \, |
\right) | \right) | ||
</math> | </math> | ||
| Línea 302: | Línea 323: | ||
<br/> | <br/> | ||
| - | < | + | <span |
| - | + | style = 'color:#00aa00'> | |
| - | </ | + | • |
| + | </span> | ||
El vector nulo es | El vector nulo es | ||
<math> | <math> | ||
| Línea 311: | Línea 333: | ||
, pues: | , pues: | ||
<math> | <math> | ||
| - | \vec{\mathbf{u}} + \vec{\mathbf{0}} = \vec{\mathbf{0}} + \vec{\mathbf{u}} = | + | \vec{\mathbf{u}} \, + \, \vec{\mathbf{0}} \, = \, \vec{\mathbf{0}} \, + \, \vec{\mathbf{u}} \, = \, |
\vec{\mathbf{u}} | \vec{\mathbf{u}} | ||
</math> | </math> | ||
| + | . Dado un punto cualquiera | ||
| + | <math> | ||
| + | P | ||
| + | </math> | ||
| + | , el vector | ||
| + | <math> | ||
| + | \stackrel{\longrightarrow}{PP} | ||
| + | </math> | ||
| + | es un representante del vector libre | ||
| + | <math> | ||
| + | \vec{\mathbf{0}} | ||
| + | </math> | ||
| + | . | ||
<br/> | <br/> | ||
| - | < | + | <span |
| - | + | style = 'color:#00aa00'> | |
| - | </ | + | • |
| + | </span> | ||
El vector opuesto de | El vector opuesto de | ||
<math> | <math> | ||
| Línea 330: | Línea 366: | ||
, pues: | , pues: | ||
<math> | <math> | ||
| - | \vec{\mathbf{u}} + \left( -\vec{\mathbf{u}} \right) = \left( -\vec{\mathbf{u}} \right) + | + | \vec{\mathbf{u}} \, + \, \left( -\vec{\mathbf{u}} \right) \, = \, \left( |
| - | \vec{\mathbf{u}} = \vec{\mathbf{0}} | + | -\vec{\mathbf{u}} \right) \, + \, \vec{\mathbf{u}} \, = \, \vec{\mathbf{0}} |
</math> | </math> | ||
<br/> | <br/> | ||
| - | < | + | <span |
| - | + | style = 'color:#00aa00'> | |
| - | </ | + | • |
| + | </span> | ||
Conmutativa: | Conmutativa: | ||
<math> | <math> | ||
| - | \vec{\mathbf{u}} + \vec{\mathbf{v}} = \vec{\mathbf{v}} + \vec{\mathbf{u}} | + | \vec{\mathbf{u}} \, + \, \vec{\mathbf{v}}\, = \,\vec{\mathbf{v}} \, + \, \vec{\mathbf{u}} |
</math> | </math> | ||
| Línea 364: | Línea 401: | ||
como un nuevo vector que tiene por módulo el producto | como un nuevo vector que tiene por módulo el producto | ||
<math> | <math> | ||
| - | \left| \, \alpha | + | \left| \, \alpha \, \right| \cdot \left| \, \vec{\mathbf{u}} \, \right| |
</math> | </math> | ||
, por dirección la misma de | , por dirección la misma de | ||
| Línea 398: | Línea 435: | ||
\alpha | \alpha | ||
\left( | \left( | ||
| - | \, \vec{\mathbf{u}} + \vec{\mathbf{v}} \, | + | \, \vec{\mathbf{u}} \, + \, \vec{\mathbf{v}} \, |
\right) | \right) | ||
| - | = \alpha \vec{\mathbf{u}} + \alpha \vec{\mathbf{v}} | + | \, = \, \alpha \vec{\mathbf{u}} \, + \, \alpha \vec{\mathbf{v}} |
</math> | </math> | ||
</center> | </center> | ||
| Línea 409: | Línea 446: | ||
<math> | <math> | ||
\left( | \left( | ||
| - | \, \alpha + \mu \, | + | \, \alpha \, + \, \mu \, |
\right) | \right) | ||
\vec{\mathbf{u}} | \vec{\mathbf{u}} | ||
| - | = \alpha \vec{\mathbf{u}} + \mu \vec{\mathbf{u}} | + | \, = \, \alpha \vec{\mathbf{u}} \, + \, \mu \vec{\mathbf{u}} |
</math> | </math> | ||
</center> | </center> | ||
| Línea 420: | Línea 457: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | 1 \cdot \vec{\mathbf{u}} = \vec{\mathbf{u}} | + | 1 \cdot \vec{\mathbf{u}} \, = \, \vec{\mathbf{u}} |
</math> | </math> | ||
</center> | </center> | ||
| Línea 431: | Línea 468: | ||
\left( | \left( | ||
\, \mu \vec{\mathbf{u}} \, | \, \mu \vec{\mathbf{u}} \, | ||
| - | \right) = | + | \right) \, = \, |
\left( | \left( | ||
\, \alpha \mu \, | \, \alpha \mu \, | ||
| Línea 443: | Línea 480: | ||
Además, si | Además, si | ||
<math> | <math> | ||
| - | \alpha \vec{\mathbf{u}} = \vec{\mathbf{0}} | + | \alpha \vec{\mathbf{u}} \, = \, \vec{\mathbf{0}} |
</math> | </math> | ||
, se verifica que, o bien | , se verifica que, o bien | ||
<math> | <math> | ||
| - | \alpha = 0 | + | \alpha \, = \, 0 |
</math> | </math> | ||
o bien | o bien | ||
<math> | <math> | ||
| - | \vec{\mathbf{u}} = \vec{\mathbf{0}} | + | \vec{\mathbf{u}} \, = \, \vec{\mathbf{0}} |
</math> | </math> | ||
. | . | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Un '''''espacio vectorial''''' es un conjunto ( de vectores ) donde se define una operacion suma y una | ||
| + | operacion producto por un numero real y estas operaciones satisfacen las propiedades de la | ||
| + | suma y producto por un numero real que hemos visto en el conjunto de los vectores libres | ||
| + | del plano. | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | De hecho, en la definicion de espacio vectorial que acabamos de ver los numeros reales | ||
| + | pueden ser sustituidos por otro conjunto, como el conjunto de los numeros complejos, pero | ||
| + | a nivel de bachillerato ( wikillerato ) nos centraremos en los numeros reales. | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | ====Ejemplo==== | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | El conjunto | ||
| + | <math> | ||
| + | R^3 | ||
| + | </math> | ||
| + | se define como el conjunto de ternas | ||
| + | <math> | ||
| + | \left( | ||
| + | \, x, \, y, \, z \, | ||
| + | \right) | ||
| + | </math> | ||
| + | de números reales. En | ||
| + | <math> | ||
| + | R^3 | ||
| + | </math> | ||
| + | se definen la suma y el producto por un número real así: | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | <span | ||
| + | style= 'color:#00AA00'> | ||
| + | 1. | ||
| + | <span> | ||
| + | Suma: | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | <center> | ||
| + | <math> | ||
| + | \left( | ||
| + | \, x_1, \, y_1, \, z_1 \, | ||
| + | \right) | ||
| + | \, + \, | ||
| + | \left( | ||
| + | \, x_2, \, y_2, \, z_2 \, | ||
| + | \right) | ||
| + | \, = \, | ||
| + | \left( | ||
| + | \, x_1 \, + \, x_2, \, y_1 \, + \, y_2, \, z_1 \, + \, z_2 \, | ||
| + | \right) | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | <span | ||
| + | style= 'color:#00AA00'> | ||
| + | 2. | ||
| + | <span> | ||
| + | Producto por un número real: | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | <center> | ||
| + | <math> | ||
| + | \alpha | ||
| + | \left( | ||
| + | \, x, \, y, \, z \, | ||
| + | \right) | ||
| + | \, = \, | ||
| + | \left( | ||
| + | \, \alpha x, \, \alpha y, \, \alpha z \, | ||
| + | \right) | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | El conjunto | ||
| + | <math> | ||
| + | R^3 | ||
| + | </math> | ||
| + | con estas operaciones es un espacio vectorial. | ||
<br/> | <br/> | ||
[[Category:Matemáticas]] | [[Category:Matemáticas]] | ||
Revisión actual
Tabla de contenidos |
Vectores en el plano
En el plano, un vector fijo
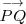 es un segmento orientado de origen
es un segmento orientado de origen
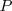 y extremo
y extremo
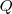 , que tiene las siguientes caracteristicas:
, que tiene las siguientes caracteristicas:
•
Módulo: longitud del segmento
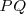 .
.
• Dirección: la de la recta que lo contiene y todas sus paralelas.
• Sentido: el que va del origen al extremo.
Los vectores
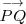 y
y
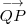 tienen el mismo módulo y la misma dirección, pero sentido contrario.
Los vectores
tienen el mismo módulo y la misma dirección, pero sentido contrario.
Los vectores
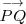 y
y
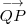 son opuestos.
son opuestos.
El conjunto de todos los vectores fijos del mismo módulo, dirección y sentido forma lo
que se denomina un vector libre. Una propiedad importante que cumplen los vectores libres
es que si
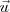 es un vector libre y
es un vector libre y
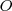 es un punto del plano, existe un único punto
es un punto del plano, existe un único punto
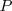 tal que
tal que
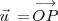 .
.
Componentes de un vector
Un sistema de referencia esta formado por dos rectas
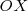 y
y
 , llamadas ejes de coordenadas que se cortan en un punto
, llamadas ejes de coordenadas que se cortan en un punto
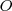 , origen de coordenadas, y una unidad de medida en cada eje. Cuando las dos rectas
son perpendiculares el sistema es ortogonal y cuando, además, las dos unidades de medida
son iguales a uno, el sistema es ortonormal.
, origen de coordenadas, y una unidad de medida en cada eje. Cuando las dos rectas
son perpendiculares el sistema es ortogonal y cuando, además, las dos unidades de medida
son iguales a uno, el sistema es ortonormal.
Para representar un punto
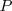 del plano en un sistema de coordenadas cartesiano
se trazan desde
del plano en un sistema de coordenadas cartesiano
se trazan desde
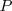 perpendiculares a los ejes, obteniendo
perpendiculares a los ejes, obteniendo
 y
y
 . Si la distancia de
. Si la distancia de
 a
a
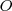 es
es
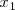 , y la de
, y la de
 a
a
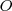 es
es
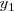 , entonces
, entonces
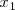 e
e
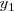 reciben el nombre de coordenadas del punto
reciben el nombre de coordenadas del punto
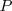 . Se escribe
. Se escribe
 , siendo
, siendo
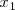 la abcisa e
la abcisa e
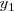 la ordenada.
la ordenada.
Conocidas las coordenadas del origen
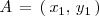 y del extremo
y del extremo
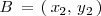 de un vector fijo
de un vector fijo
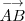 , se puede determinar las componentes del vector restando a las coordenadas del
extremo las del origen:
, se puede determinar las componentes del vector restando a las coordenadas del
extremo las del origen:
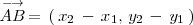
Suma de vectores
Sean
 y
y
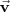 dos vectores libres, se define el vector suma
dos vectores libres, se define el vector suma
 como otro vector obtenido de la siguiente forma:
como otro vector obtenido de la siguiente forma:
1.
Se señala un punto
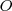 del plano y se traza el vector
del plano y se traza el vector
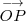 representante de
representante de
 .
.
2.
Por el extremo
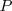 se traza el vector
se traza el vector
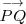
3.
El vector
 que tiene como origen
que tiene como origen
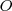 ( origen del primero ) y como extremo
( origen del primero ) y como extremo
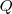 ( extremo del segundo ) es el representante del vector suma
( extremo del segundo ) es el representante del vector suma
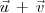 .
.
La suma tiene las siguientes propiedades:
•
Asociativa:
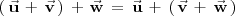
•
El vector nulo es
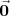 , pues:
, pues:
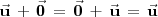 . Dado un punto cualquiera
. Dado un punto cualquiera
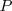 , el vector
, el vector
 es un representante del vector libre
es un representante del vector libre
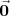 .
.
•
El vector opuesto de
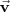 es
es
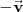 , pues:
, pues:
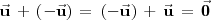
•
Conmutativa:

Producto de un número real por un vector
Si
 es un vector libre y
es un vector libre y
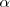 un número real, se define el producto
un número real, se define el producto
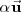 como un nuevo vector que tiene por módulo el producto
como un nuevo vector que tiene por módulo el producto
 , por dirección la misma de
, por dirección la misma de
 y sentido el mismo de
y sentido el mismo de
 si
si
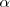 es positivo, y opuesto, si
es positivo, y opuesto, si
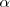 es negativo.
es negativo.
El producto de un número real por un vector tiene las siguientes propiedades:

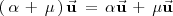
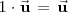
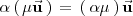
Además, si
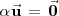 , se verifica que, o bien
, se verifica que, o bien
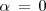 o bien
o bien
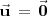 .
.
Un espacio vectorial es un conjunto ( de vectores ) donde se define una operacion suma y una operacion producto por un numero real y estas operaciones satisfacen las propiedades de la suma y producto por un numero real que hemos visto en el conjunto de los vectores libres del plano.
De hecho, en la definicion de espacio vectorial que acabamos de ver los numeros reales pueden ser sustituidos por otro conjunto, como el conjunto de los numeros complejos, pero a nivel de bachillerato ( wikillerato ) nos centraremos en los numeros reales.
Ejemplo
El conjunto
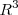 se define como el conjunto de ternas
se define como el conjunto de ternas
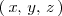 de números reales. En
de números reales. En
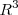 se definen la suma y el producto por un número real así:
se definen la suma y el producto por un número real así:
1. Suma:
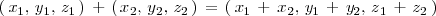
2. Producto por un número real:
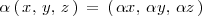
El conjunto
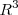 con estas operaciones es un espacio vectorial.
con estas operaciones es un espacio vectorial.





