De Wikillerato
(Diferencias entre revisiones)
|
|
| Línea 1: |
Línea 1: |
| - | Una función
| |
| - | <math>
| |
| - | \mathrm{f}
| |
| - | </math>
| |
| - | es continua en el punto
| |
| - | <math>
| |
| - | x \, = \, x_0
| |
| - | </math>
| |
| - | si
| |
| - | <math>
| |
| - | \lim_{x \to x_0} \mathrm{f} \left( \, x \, \right) \, = \,
| |
| - | \mathrm{f} \left( \, x_0 \, \right)
| |
| - | </math>.
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | El que una función
| |
| - | <math>
| |
| - | \mathrm{f}
| |
| - | </math>
| |
| - | sea continua en el punto
| |
| - | <math>
| |
| - | x \, = \, x_0
| |
| - | </math>
| |
| - | implica que
| |
| - | <math>
| |
| - | \mathrm{f} \left( \, x_0 \, \right)
| |
| - | </math>
| |
| - | existe y que
| |
| - | <math>
| |
| - | \lim_{x \to x_0} \mathrm{f} \left( \, x \, \right)
| |
| - | </math>
| |
| - | tambien existe.
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | Una función es continua en un intervalo si es continua en todos los puntos del intervalo.
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | Una función es continua en todo su dominio cuando lo es en todos los puntos que lo
| |
| - | componen.
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | [[Category:Matemáticas]]
| |
| - |
| |
| - | %% }}}
| |
| - | %% {{{ =discontinuidades
| |
| - |
| |
| | Una función es '''''discontinua''''' en un punto | | Una función es '''''discontinua''''' en un punto |
| | <math> | | <math> |
Revisión de 14:10 11 ene 2007
Una función es discontinua en un punto
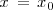 si
si
 no es continua en dicho punto.
no es continua en dicho punto.
Una función
 tiene una discontinuidad evitable en un punto
tiene una discontinuidad evitable en un punto
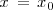 cuando existe el limite de la función en dicho punto.
cuando existe el limite de la función en dicho punto.
Ejemplo
La función
 definida por:
definida por:
![\mathrm{f} \left( \, x \, \right) \, = \,
\left\{
</p>
<pre> \begin{array}[c]{ll}
\frac{x^2 \, - \, 1}{x \, + \, 1}, & \qquad \makebox{si} x \neq 1
\\
0, & \qquad \makebox{si} x \, = \, 1
\end{array}
</pre>
<p>\right.
\mathrm{f} \left( \, x \, \right) \, = \,
\left\{
</p>
<pre> \begin{array}[c]{ll}
\frac{x^2 \, - \, 1}{x \, + \, 1}, & \qquad \makebox{si} x \neq 1
\\
0, & \qquad \makebox{si} x \, = \, 1
\end{array}
</pre>
<p>\right.](/images/math/math-8880f2c6ed8b366d29b0787616ecc4c2.png)
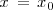 si
si
 no es continua en dicho punto.
no es continua en dicho punto.
 tiene una discontinuidad evitable en un punto
tiene una discontinuidad evitable en un punto
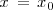 cuando existe el limite de la función en dicho punto.
cuando existe el limite de la función en dicho punto.
 definida por:
definida por:
![\mathrm{f} \left( \, x \, \right) \, = \,
\left\{
</p>
<pre> \begin{array}[c]{ll}
\frac{x^2 \, - \, 1}{x \, + \, 1}, & \qquad \makebox{si} x \neq 1
\\
0, & \qquad \makebox{si} x \, = \, 1
\end{array}
</pre>
<p>\right.
\mathrm{f} \left( \, x \, \right) \, = \,
\left\{
</p>
<pre> \begin{array}[c]{ll}
\frac{x^2 \, - \, 1}{x \, + \, 1}, & \qquad \makebox{si} x \neq 1
\\
0, & \qquad \makebox{si} x \, = \, 1
\end{array}
</pre>
<p>\right.](/images/math/math-8880f2c6ed8b366d29b0787616ecc4c2.png)



