|
|
| Línea 1: |
Línea 1: |
| - | Un '''''sistema de ecuaciones lineales''''' con incógnitas
| + | __TOC__ |
| - | <math>
| + | |
| - | \left(
| + | |
| - | \, x_1, \, x_2, \, \ldots, \, x_n \,
| + | |
| - | \right)
| + | |
| - | </math>
| + | |
| - | es un conjunto formado por
| + | |
| - | <math>
| + | |
| - | m
| + | |
| - | </math>
| + | |
| - | igualdades de la forma:
| + | |
| | | | |
| | <br/> | | <br/> |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | \left.
| |
| - | \begin{array}{c}
| |
| - | a_{11} \cdot x_1 + a_{12} \cdot x_2 + \ldots a_{1n} \cdot x_n = b_1
| |
| - | \\
| |
| - | a_{21} \cdot x_1 + a_{22} \cdot x_2 + \ldots a_{2n} \cdot x_n = b_2
| |
| - | \\
| |
| - | \dotfill
| |
| - | \\
| |
| - | a_{m1} \cdot x_1 + a_{m2} \cdot x_2 + \ldots a_{mn} \cdot x_n = b_m
| |
| - | \end{array}
| |
| - | \right\}
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | donde los
| |
| - | <math>
| |
| - | a_{ij}
| |
| - | </math>
| |
| - | se llaman coeficientes y los
| |
| - | <math>
| |
| - | b_i
| |
| - | </math>
| |
| - | , terminos independientes del sistema.
| |
| - |
| |
| - | En los coeficientes
| |
| - | <math>
| |
| - | a_{ij}
| |
| - | </math>
| |
| - | , el subindice
| |
| - | <math>
| |
| - | i
| |
| - | </math>
| |
| - | indica la ecuación del sistema en la que aparece dicho coeficiente, y el subíndice
| |
| - |
| |
| - | <math>
| |
| - | j
| |
| - | </math>
| |
| - | señala de que incognita es coeficiente
| |
| - | <math>
| |
| - | a_{ij}
| |
| - | </math>
| |
| - | .
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | El subindice
| |
| - | <math>
| |
| - | i
| |
| - | </math>
| |
| - | que aparece en el término
| |
| - | <math>
| |
| - | b_i
| |
| - | </math>
| |
| - | , indica la ecuación de la que
| |
| - | <math>
| |
| - | b_i
| |
| - | </math>
| |
| - | es término independiente.
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | El sistema anterior de
| |
| - | <math>
| |
| - | m
| |
| - | </math>
| |
| - | ecuaciones lineales con
| |
| - | <math>
| |
| - | n
| |
| - | </math>
| |
| - | incognitas se puede escribir matricialmente de la siguiente forma:
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | \left(
| |
| - | \begin{array}[c]{cccc}
| |
| - | a_{11} & a_{12} & \ldots & a_{1n}
| |
| - | \\
| |
| - | a_{21} & a_{22} & \ldots & a_{2n}
| |
| - | \\
| |
| - | \vdots & \vdots & \ddots & \vdots
| |
| - | \\
| |
| - | a_{m1} & a_{m2} & \ldots & a_{mn}
| |
| - | \end{array}
| |
| - | \right)
| |
| - | \cdot
| |
| - | \left(
| |
| - | \begin{array}[c]{c}
| |
| - | x_1
| |
| - | \\
| |
| - | x_2
| |
| - | \\
| |
| - | \vdots
| |
| - | \\
| |
| - | x_n
| |
| - | \end{array}
| |
| - | \right)
| |
| - | \, = \,
| |
| - | \left(
| |
| - | \begin{array}[c]{c}
| |
| - | b_1
| |
| - | \\
| |
| - | b_2
| |
| - | \\
| |
| - | \vdots
| |
| - | \\
| |
| - | b_m
| |
| - | \end{array}
| |
| - | \right)
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | De izquierda a derecha, la primera matriz, en la igualdad anterior es la '''''matriz de los coeficientes''''' y la llamaremos
| |
| - |
| |
| - | <math>
| |
| - | A
| |
| - | </math>
| |
| - | , la segunda matriz es la matriz de las incognitas y la llamaremos
| |
| - | <math>
| |
| - | X
| |
| - | </math>
| |
| - | . La tercera es la matriz de los terminos indedependientes y la llamaremos
| |
| - | <math>
| |
| - | B
| |
| - | </math>
| |
| - | .
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | Con esta notación, nuestro sistema de ecuaciones lineales se puede representar de la
| |
| - | siguiente manera:
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | A \cdot X \, = \, B
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | La
| |
| - | '''''matriz ampliada''''' es la matriz de los coeficientes,
| |
| - | <math>
| |
| - | A
| |
| - | </math>
| |
| - | , a la que se añade la columna de los terminos independientes,
| |
| - | <math>
| |
| - | B
| |
| - | </math>
| |
| - | :
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | A|B \, = \,
| |
| - | \left(
| |
| - | \left.
| |
| - | \begin{array}[c]{cccc}
| |
| - | a_{11} & a_{12} & \ldots & a_{1n}
| |
| - | \\
| |
| - | a_{21} & a_{22} & \ldots & a_{2n}
| |
| - | \\
| |
| - | \vdots & \vdots & \ddots & \vdots
| |
| - | \\
| |
| - | a_{m1} & a_{m2} & \ldots & a_{mn}
| |
| - | \end{array}
| |
| - | \right|
| |
| - | \begin{array}[c]{c}
| |
| - | b_1
| |
| - | \\
| |
| - | b_2
| |
| - | \\
| |
| - | \vdots
| |
| - | \\
| |
| - | b_m
| |
| - | \end{array}
| |
| - | \right)
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | Resolver un sistema de ecuaciones lineales es encontrar todas sus soluciones. Al conjunto
| |
| - | de todas las soluciones del sistema se le llama solución general, y a cada una de las
| |
| - | soluciones que forman dicho conjunto, solución particular.
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | Serán soluciones del sistema todas las n-tuplas
| |
| - | <math>
| |
| - | \left(
| |
| - | \, s_1, \, s_2, \, \ldots, \, s_n \,
| |
| - | \right)
| |
| - | </math>
| |
| - | tales que al sustituir
| |
| - | <math>
| |
| - | x_i
| |
| - | </math>
| |
| - | por
| |
| - | <math>
| |
| - | s_i
| |
| - | </math>
| |
| - | , para
| |
| - | <math>
| |
| - | i = 1, \, 2, \, \ldots, \, n
| |
| - | </math>
| |
| - | , todas las ecuaciones del sistema se conviertan en identidades.
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | [[Categoría:Matemáticas]]
| |
| - |
| |
| - | %% }}}
| |
| - | %% {{{ =tipos de sistemas
| |
| - | <center>
| |
| - | [[Image:sistemas.gif|Clasificación de los sistemas de ecuaciones lineales]]
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | Un sistema de ecuaciones es '''''compatible''''' cuando tiene solución.
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | Un sistema de ecuaciones es '''''compatible determinado''''' cuando tiene solución única.
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | Un sistema de ecuaciones es '''''compatible indeterminado''''' cuando tiene infinitas
| |
| - | soluciones.
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | Un sistema de ecuaciones es '''''incompatible''''' cuando no tiene solución.
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | Un sistema de ecuaciones es '''''homogéneo''''' cuando todos sus terminos independientes
| |
| - | son cero.
| |
| - |
| |
| - | [[Category:Matemáticas]]
| |
| - | %% }}}
| |
| - | %% {{{ =método de reducción de Gauss
| |
| - |
| |
| - | [[Imagen:gauss.jpg|frame|Gauss es uno de los matematicos mas
| |
| - | importantes de todos los tiempos. ¡Fue un GENIO!]]
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | El método de Gauss consiste en transformar el sistema dado en otro equivalente.
| |
| - | Para ello tomamos la [[Sistemas de ecuaciones lineales|matriz ampliada]] del
| |
| - | sistema y mediante las [[Matriz inversa#Operaciones elementales por filas en una matriz|operaciones elementales]]
| |
| - | por filas la transformamos en una matriz triangular superior ( o
| |
| - | inferior ). De esta forma obtenemos un sistema equivalente al inicial y que es muy facil
| |
| - | de resolver.
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | ==Ejemplo==
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | La matriz ampliada del sistema de ecuaciones:
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | \left\{
| |
| - | \begin{array}[c]{ccc}
| |
| - | x \, + \, y \, + \, z & = & ~~3
| |
| - | \\
| |
| - | x \, + \, y \, - \, z & = & ~~1
| |
| - | \\
| |
| - | x \, - \, y \, - \, z & = & -1
| |
| - | \end{array}
| |
| - | \right.
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | es:
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | \left(
| |
| - | \left.
| |
| - | \begin{array}[c]{ccc}
| |
| - | ~~1 & ~~1 & ~~1
| |
| - | \\
| |
| - | ~~1 & ~~1 & -1
| |
| - | \\
| |
| - | ~~1 & -1 & -1
| |
| - | \end{array}
| |
| - | \right|
| |
| - | \begin{array}[c]{c}
| |
| - | ~~3
| |
| - | \\
| |
| - | ~~1
| |
| - | \\
| |
| - | -1
| |
| - | \end{array}
| |
| - | \right)
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | Si a la tercera y segunda fila le restamos la primera, obtenemos:
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | \left(
| |
| - | \left.
| |
| - | \begin{array}[c]{ccc}
| |
| - | ~~1 & ~~1 & ~~1
| |
| - | \\
| |
| - | ~~0 & ~~0 & -2
| |
| - | \\
| |
| - | ~~0 & -2 & -2
| |
| - | \end{array}
| |
| - | \right|
| |
| - | \begin{array}[c]{c}
| |
| - | ~~3
| |
| - | \\
| |
| - | -2
| |
| - | \\
| |
| - | -4
| |
| - | \end{array}
| |
| - | \right)
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | Si ahora intercambiamos la segunda y tercera filas, obtenemos
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | \left(
| |
| - | \left.
| |
| - | \begin{array}[c]{ccc}
| |
| - | ~~1 & ~~1 & ~~1
| |
| - | \\
| |
| - | ~~0 & -2 & -2
| |
| - | \\
| |
| - | ~~0 & ~~0 & -2
| |
| - | \end{array}
| |
| - | \right|
| |
| - | \begin{array}[c]{c}
| |
| - | ~~3
| |
| - | \\
| |
| - | -4
| |
| - | \\
| |
| - | -2
| |
| - | \end{array}
| |
| - | \right)
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | que es la matriz ampliada del sistema de ecuaciones:
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | \left\{
| |
| - | \begin{array}[c]{rcl}
| |
| - | x \, + \, y \, + \, z & = & ~~3
| |
| - | \\
| |
| - | -2y \, - \, 2z & = & -4
| |
| - | \\
| |
| - | -2z & = & -2
| |
| - | \end{array}
| |
| - | \right.
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | que es equivalente al inicial.
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | Solucionamos la tercera ocuacion para obtener
| |
| - | <math>
| |
| - | z
| |
| - | </math>
| |
| - | :
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | z \, = \, 1
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - | En la primera y segunda ecuación, sustituimos
| |
| - | <math>
| |
| - | z
| |
| - | </math>
| |
| - | por la solucion de la tercera ecuación (
| |
| - | <math>
| |
| - | 1 \to z
| |
| - | </math>
| |
| - | ), para obtener:
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | \left\{
| |
| - | \begin{array}[c]{rcl}
| |
| - | x \, + \, y \, + \, 1 & = & ~~3
| |
| - | \\
| |
| - | -2y \, - \, 2 & = & -4
| |
| - | \end{array}
| |
| - | \right.
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | La segunda ecuación es ahora una ecuación con una sola incognita,
| |
| - | <math>
| |
| - | y
| |
| - | </math>
| |
| - | . Que resolvemos para obtener
| |
| - | <math>
| |
| - | y \, = \, 1
| |
| - | </math>
| |
| - | . Sustituimos, en la primera ecuación,
| |
| - | <math>
| |
| - | y
| |
| - | </math>
| |
| - | por 1 (
| |
| - | <math>
| |
| - | 1 \to y
| |
| - | </math>
| |
| - | ). Esto nos da una ecuación en
| |
| - | <math>
| |
| - | x
| |
| - | </math>
| |
| - | :
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | x \, + \, 1 \, + \, 1 \, = \, 3
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | que al resolverla termina de darnos la solución del sistema de ecuaciones inicial:
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | x \, = \, y \, = \, z \, = \, 1
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | [[Category:Matemáticas]]
| |
| - |
| |
| - | %% }}}
| |
| - | %% {{{ =método de la matriz inversa
| |
| - |
| |
| - | Un sistema de ecuaciones lineales se puede escribir en [[Sistemas de ecuaciones lineales|forma matricial]]:
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | A \cdot X \, = \, B
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | Si
| |
| - | <math>
| |
| - | A^{-1}
| |
| - | </math>
| |
| - | existe, es decir, si
| |
| - | <math>
| |
| - | A
| |
| - | </math>
| |
| - | es una matriz cuadrada de determinante no nulo, entonces podemos multiplicar toda
| |
| - | la igualdad anterior por la izquierda por
| |
| - | <math>
| |
| - | A^{-1}
| |
| - | </math>
| |
| - | , para obtener:
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | X \, = \, A^{-1} \cdot B
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | que es la solución del sistema de ecuaciones lineales de matriz de coeficientes
| |
| - | <math>
| |
| - | A
| |
| - | </math>
| |
| - | y matriz de terminos independientes
| |
| - | <math>
| |
| - | B
| |
| - | </math>
| |
| - | .
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | [[Category:Matemáticas]]
| |
| - |
| |
| - | %% }}}
| |
| - | %% {{{ =regla de Cramer
| |
| - |
| |
| - | [[Imagen:cramer2.gif|frame|Gabriel Cramer nacio Ginebra ( Suiza ) 1704 y murio en 1752. A
| |
| - | él le debemos la regla que lleva su nombre. ¡Gracias Gabriel por tu contribución a las Matemáticas!]]
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | Esta regla es un metodo de resolución de sistemas de ecuaciones lineales que se puede
| |
| - | utilizar cuando la matriz
| |
| - | <math>
| |
| - | A
| |
| - | </math>
| |
| - | de coeficientes del sistema es cuadrada y de determinante no nulo. El que
| |
| - | <math>
| |
| - | A
| |
| - | </math>
| |
| - | sea cuadrada significa que el numero de incognitas y el numero de ecuaciones
| |
| - | coincide.
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | Cuando el sistema de ecuaciones
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | \left.
| |
| - | \begin{array}{c}
| |
| - | a_{11} \cdot x_1 + a_{12} \cdot x_2 + \ldots a_{1n} \cdot x_n = b_1
| |
| - | \\
| |
| - | a_{21} \cdot x_1 + a_{22} \cdot x_2 + \ldots a_{2n} \cdot x_n = b_2
| |
| - | \\
| |
| - | \dotfill
| |
| - | \\
| |
| - | a_{m1} \cdot x_1 + a_{m2} \cdot x_2 + \ldots a_{mn} \cdot x_n = b_m
| |
| - | \end{array}
| |
| - | \right\}
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | satisface las condiciones arriba mencionadas, su solución viene dada por:
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | x_1 \, = \, \frac
| |
| - | {
| |
| - | \left|
| |
| - | \begin{array}[c]{cccc}
| |
| - | b_1 & a_{12} & \ldots & a_{1n}
| |
| - | \\
| |
| - | b_2 & a_{22} & \ldots & a_{2n}
| |
| - | \\
| |
| - | \vdots & \vdots & \ddots & \vdots
| |
| - | \\
| |
| - | b_m & a_{m2} & \ldots & a_{mn}
| |
| - | \end{array}
| |
| - | \right|
| |
| - | }
| |
| - | {|A|}
| |
| - | , \qquad \qquad x_2 \, = \, \frac
| |
| - | {
| |
| - | \left|
| |
| - | \begin{array}[c]{cccc}
| |
| - | a_{11} & b_1 & \ldots & a_{1n}
| |
| - | \\
| |
| - | a_{21} & b_2 & \ldots & a_{2n}
| |
| - | \\
| |
| - | \vdots & \vdots & \ddots & \vdots
| |
| - | \\
| |
| - | a_{m1} & b_m & \ldots & a_{mn}
| |
| - | \end{array}
| |
| - | \right|
| |
| - | }
| |
| - | {|A|}, \qquad \qquad \ldots \ldots
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | \ldots \ldots, \qquad \qquad x_n \, = \, \frac
| |
| - | {
| |
| - | \left|
| |
| - | \begin{array}[c]{cccc}
| |
| - | a_{11} & a_{12} & \ldots & b_1
| |
| - | \\
| |
| - | a_{21} & a_{22} & \ldots & b_2
| |
| - | \\
| |
| - | \vdots & \vdots & \ddots & \vdots
| |
| - | \\
| |
| - | a_{m1} & a_{m2} & \ldots & b_m
| |
| - | \end{array}
| |
| - | \right|
| |
| - | }
| |
| - | {|A|}
| |
| - | \qquad \qquad
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | En general
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | x_i \, = \, \frac{|A_i|}{|A|}
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | donde
| |
| - | <math>
| |
| - | A_i
| |
| - | </math>
| |
| - | es la matriz que se obtiene sustituyendo la i-esima columna de
| |
| - | <math>
| |
| - | A
| |
| - | </math>
| |
| - | por la [[Sistemas de ecuaciones lineales|matriz de los terminos independientes]],
| |
| - | <math>
| |
| - | B
| |
| - | </math>
| |
| - | .
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | ==Ejemplo==
| |
| - |
| |
| - | <br/>
| |
| - | Consideremos el sistema de ecuaciones:
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | \left\{
| |
| - | \begin{array}[c]{rcl}
| |
| - | x \, + \, y \, = \, 2
| |
| - | \\
| |
| - | x \, - \, y \, = \, 0
| |
| - | \end{array}
| |
| - | \right.
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | En este sistema de ecuaciones lineales, la matriz
| |
| - | <math>
| |
| - | A
| |
| - | </math>
| |
| - | de los coeficientes es una matriz cuadrada y
| |
| - | <math>
| |
| - | |A| \, = \,
| |
| - | \left|
| |
| - | \begin{array}[c]{cc}
| |
| - | 1 & ~~1
| |
| - | \\
| |
| - | 1 & -1
| |
| - | \end{array}
| |
| - | \right|
| |
| - | \, = \, -2 \neq 0
| |
| - | </math>
| |
| - | . Por lo tanto, podemos aplicar la regla de Cramer para resolverlo:
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | <center>
| |
| - | <math>
| |
| - | x \, = \, \frac
| |
| - | {
| |
| - | \left|
| |
| - | \begin{array}[c]{cc}
| |
| - | 2 & ~~1
| |
| - | \\
| |
| - | 0 & -1
| |
| - | \end{array}
| |
| - | \right|
| |
| - | }
| |
| - | {|A|} \, = \, \frac{-2}{-2} \, = \, 1
| |
| - | \qquad \qquad y \, = \, \frac
| |
| - | {
| |
| - | \left|
| |
| - | \begin{array}[c]{cc}
| |
| - | 1 & 2
| |
| - | \\
| |
| - | 1 & 0
| |
| - | \end{array}
| |
| - | \right|
| |
| - | }
| |
| - | {|A|}\, = \, \frac{-2}{-2} \, = \, 1
| |
| - | </math>
| |
| - | </center>
| |
| - |
| |
| - | <br/>
| |
| - |
| |
| - | [[Category:Matemáticas]]
| |
| - |
| |
| - | %% }}}
| |
| - | %% {{{ =teorema de Rouché-Frobenius
| |
| - |
| |
| - | __TOC__
| |
| | | | |
| | ==Enunciado== | | ==Enunciado== |
1. Que el rango de la matriz de los coeficientes sea menor que el numero de incognitas.
2. Que el rango de la matriz de los coeficientes sea igual al numero de incognitas.
Un sistema homogeneo es compatible indeterminado cuando el determinante de la matriz de
los coeficientes es cero. Si este determinante no es cero el sistema homogeneo es
compatible determinado.
Navegación
ecuaciones lineales con
incognitas es compatible ( tiene solución ) si, y sólo si, el rango de la matriz de los coeficientes coincide con el rango de la matriz
ampliada.
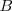de los terminos independientes es una matriz nula, de manera que el
rango de la
matriz de los coeficientes y el de la
matriz ampliada coinciden. Esto implica, a su vez, por el teorema de Rouché-Fröbenius,
que un sistema homogeneo siempre es compatible. En cualquier sistema homogeneo, siempre
podemos obtener una solución particular igualando todas las incognitas a 0.
