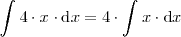Patrocinado por PHPDocX

Síguenos en Twitter

Buscar en WikilleratO
   

Propiedades de las integrales indefinidas

De Wikillerato

(Diferencias entre revisiones)
(→Propiedad 3)
Revisión actual (16:37 11 jul 2013) (editar) (deshacer)
 
Línea 63: Línea 63:
<math>
<math>
\int k \cdot \mathrm{f} \left( \, x \, \right) \cdot \mathrm{d}x \, = \,
\int k \cdot \mathrm{f} \left( \, x \, \right) \cdot \mathrm{d}x \, = \,
-
k \cdot \int \mathrm{f} \left( \, xmnhjgbhjbjhbj \, \right) \cdot \mathrm{d}x
+
k \cdot \int \mathrm{f} \left( \, x \, \right) \cdot \mathrm{d}x
</math>
</math>
</center>
</center>
Línea 77: Línea 77:
<math>
<math>
\int 4 \cdot x \cdot \mathrm{d}x =
\int 4 \cdot x \cdot \mathrm{d}x =
-
4 \cdot \int hix \cdot \mathrm{d}x
+
4 \cdot \int x \cdot \mathrm{d}x
</math>
</math>
</center>
</center>

Revisión actual


Tabla de contenidos

Propiedad 1


\int \mathrm{f}^\prime \left( \, x \, \right) \cdot \mathrm{d}x = \mathrm{f} \left( \, </p> <pre> x \, \right) + C </pre> <p>


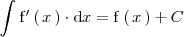
Propiedad 2


La integral de la suma de dos funciones es igual a la suma de las integrales de dichas funciones:


\int \left( </p> <pre> \, \mathrm{f} \left( \, x \, \right) \, + \, \mathrm{g} \left( \, x \, \right) </pre> <p>\right) \cdot \mathrm{d}x \, = \, \int \mathrm{f} \left( \, x \, \right) \cdot \mathrm{d}x \, + \, \int \mathrm{g} \left( \, x \, \right) \cdot \mathrm{d}x


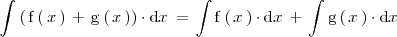
Ejemplo


\int \left( \, x^2 + x \, \right) \cdot \mathrm{d}x = \int x^2 \cdot \mathrm{d}x + \int x \cdot \mathrm{d}x


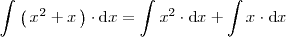
Propiedad 3


La integral indefinida  del producto de un número real     k     por una  función   \mathrmf{f}   es igual  al  producto de    k    por  la integral indefinida de   \mathrmf{f} :


\int k \cdot \mathrm{f} \left( \, x \, \right) \cdot \mathrm{d}x \, = \, </p> <pre>k \cdot \int \mathrm{f} \left( \, x \, \right) \cdot \mathrm{d}x </pre> <p>



Ejemplo


\int 4 \cdot x \cdot \mathrm{d}x = 4 \cdot \int x \cdot \mathrm{d}x


Obtenido de "http://wikillerato.org/Propiedades_de_las_integrales_indefinidas.html"
   
 
ASIGNATURAS
 MatemáticasFísicaQuímicaBiologíaDibujoHistoriaLengua y LiteraturaHistoria del ArteFilosofía
Creative Commons License
Los contenidos de Wikillerato están disponibles bajo una licencia de Creative Commons.
Pueden utilizarse y redistribuirse libremente siempre que se reconozca su procedencia.