Ángulo doble y ángulo mitad
De Wikillerato
(Diferencias entre revisiones)
| (3 ediciones intermedias no se muestran.) | |||
| Línea 33: | Línea 33: | ||
Teniendo en cuenta que | Teniendo en cuenta que | ||
<math> | <math> | ||
| - | \mathrm{sen} | + | \mathrm{sen}^2 |
\left( | \left( | ||
\, \alpha \, | \, \alpha \, | ||
\right) | \right) | ||
| - | + | \, + \, \mathrm{cos}^2 | |
\left( | \left( | ||
\, \alpha \, | \, \alpha \, | ||
\right) | \right) | ||
| - | + | \, = \, 1 | |
</math> | </math> | ||
, deducimos que: | , deducimos que: | ||
| Línea 63: | Línea 63: | ||
\, - \, | \, - \, | ||
\left( | \left( | ||
| - | \, 1 \, - \, \mathrm{cos} | + | \, 1 \, - \, \mathrm{cos}^2 |
\left( | \left( | ||
| - | + | \, \alpha \, | |
| - | \right) | + | \right) |
| - | + | \right) | |
| - | \right) | + | \, = \, 2 \cdot \mathrm{cos}^2 |
| - | \, = \, 2 \cdot \mathrm{cos}^2 | + | \left( |
| - | \left( | + | \, \alpha \, |
| - | + | ||
\right) | \right) | ||
\right) | \right) | ||
| Línea 80: | Línea 79: | ||
<br/> | <br/> | ||
| - | Según lo que se explica en la sección sobre [[Razones trigonométricas de la suma y diferencia de ángulos|razones trigonométricas de la suma y diferencia de ángulos]] | + | Según lo que se explica en la sección sobre [[Razones trigonométricas de la suma y diferencia de ángulos|razones trigonométricas de la suma y diferencia de ángulos]]: |
<br/> | <br/> | ||
| Línea 141: | Línea 140: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | \mathrm{cos} \left( \, 2 \alpha \, \right) \, = \, 2 \cdot | + | \left\{ |
| - | \mathrm{cos}^2 \left( \, \alpha \, \right) | + | \begin{array}[c]{rcl} |
| - | \, - \, 1 | + | \mathrm{cos} \left( \, 2 \alpha \, \right) \, & = & \, 2 \cdot |
| - | + | \mathrm{cos}^2 \left( \, \alpha \, \right) \, - \, 1 | |
| - | + | \\ | |
| - | + | & & | |
| - | + | \\ | |
| - | + | \mathrm{sen} \left( \, 2 \alpha \, \right) \, & = & \, 2 \cdot \mathrm{sen} | |
| - | \mathrm{sen} \left( \, 2 \alpha \, \right) \, = \, 2 \cdot \mathrm{sen} | + | \left( \, \alpha \, \right) \cdot \mathrm{cos} \left( \, \alpha \, \right) |
| - | \left( \, \alpha \, \right) \cdot \mathrm{cos} \left( \, \alpha \, \right) | + | \end{array} |
| + | \right. | ||
</math> | </math> | ||
</center> | </center> | ||
| Línea 171: | Línea 171: | ||
<math> | <math> | ||
\left\{ | \left\{ | ||
| - | \begin{array}[c]{ | + | \begin{array}[c]{rcl} |
| - | \mathrm{cos} \left( \, \alpha \, \right) \, & | + | \mathrm{cos} \left( \, \alpha \, \right) \, & = & \, 2 \cdot \mathrm{cos}^2 |
\left( | \left( | ||
\, \frac{\alpha}{2} \, | \, \frac{\alpha}{2} \, | ||
| Línea 179: | Línea 179: | ||
\, - \, 1 | \, - \, 1 | ||
\\ | \\ | ||
| - | \mathrm{sen} \left( \, \alpha \, \right) \, & | + | & & |
| + | \\ | ||
| + | \mathrm{sen} \left( \, \alpha \, \right) \, & = & \, 2 \cdot | ||
\mathrm{sen} | \mathrm{sen} | ||
\left( | \left( | ||
| Línea 196: | Línea 198: | ||
Si consideramos el anterior par de igualdades como un sistema de ecuaciones cuyas | Si consideramos el anterior par de igualdades como un sistema de ecuaciones cuyas | ||
| - | + | incógnitas son | |
<math> | <math> | ||
\mathrm{sen} | \mathrm{sen} | ||
| Línea 236: | Línea 238: | ||
<br/> | <br/> | ||
| - | En ambos casos se elige el signo de la | + | En ambos casos se elige el signo de la raíz [[Razones trigonométricas|en función de en qué cuadrante]] esté |
<math> | <math> | ||
\frac{\alpha}{2} | \frac{\alpha}{2} | ||
Revisión actual
Como se explica en la sección sobre las razones trigonométricas de la suma y diferencia de ángulos:
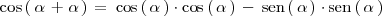
Teniendo en cuenta que
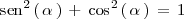 , deducimos que:
, deducimos que:
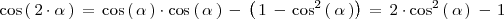
Según lo que se explica en la sección sobre razones trigonométricas de la suma y diferencia de ángulos:
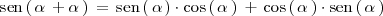
Por tanto
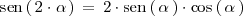
Si en las dos igualdades obtenidas:
![\left\{
</p>
<pre> \begin{array}[c]{rcl}
\mathrm{cos} \left( \, 2 \alpha \, \right) \, & = & \, 2 \cdot
\mathrm{cos}^2 \left( \, \alpha \, \right) \, - \, 1
\\
& &
\\
\mathrm{sen} \left( \, 2 \alpha \, \right) \, & = & \, 2 \cdot \mathrm{sen}
\left( \, \alpha \, \right) \cdot \mathrm{cos} \left( \, \alpha \, \right)
\end{array}
</pre>
<p>\right.
\left\{
</p>
<pre> \begin{array}[c]{rcl}
\mathrm{cos} \left( \, 2 \alpha \, \right) \, & = & \, 2 \cdot
\mathrm{cos}^2 \left( \, \alpha \, \right) \, - \, 1
\\
& &
\\
\mathrm{sen} \left( \, 2 \alpha \, \right) \, & = & \, 2 \cdot \mathrm{sen}
\left( \, \alpha \, \right) \cdot \mathrm{cos} \left( \, \alpha \, \right)
\end{array}
</pre>
<p>\right.](/images/math/math-47a3991f343b463fc7f3144fc2aee9a8.png)
sustituimos
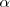 por
por
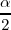 , obtenemos:
, obtenemos:
![\left\{
</p>
<pre> \begin{array}[c]{rcl}
\mathrm{cos} \left( \, \alpha \, \right) \, & = & \, 2 \cdot \mathrm{cos}^2
\left(
\, \frac{\alpha}{2} \,
\right)
\right)
\, - \, 1
\\
& &
\\
\mathrm{sen} \left( \, \alpha \, \right) \, & = & \, 2 \cdot
\mathrm{sen}
\left(
\, \frac{\alpha}{2} \,
\right)
\cdot \mathrm{cos}
\left(
\, \frac{\alpha}{2} \,
\right)
</pre>
<p>\end{array}
\right.
\left\{
</p>
<pre> \begin{array}[c]{rcl}
\mathrm{cos} \left( \, \alpha \, \right) \, & = & \, 2 \cdot \mathrm{cos}^2
\left(
\, \frac{\alpha}{2} \,
\right)
\right)
\, - \, 1
\\
& &
\\
\mathrm{sen} \left( \, \alpha \, \right) \, & = & \, 2 \cdot
\mathrm{sen}
\left(
\, \frac{\alpha}{2} \,
\right)
\cdot \mathrm{cos}
\left(
\, \frac{\alpha}{2} \,
\right)
</pre>
<p>\end{array}
\right.](/images/math/math-4f1fd31eefb8f6e79683f34e58743c08.png)
Si consideramos el anterior par de igualdades como un sistema de ecuaciones cuyas
incógnitas son
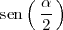 y
y
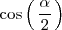 y resolvemos ese sistema de ecuaciones, se llega a las siguientes igualdades:
y resolvemos ese sistema de ecuaciones, se llega a las siguientes igualdades:
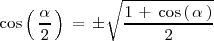
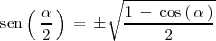
En ambos casos se elige el signo de la raíz en función de en qué cuadrante esté
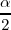 .
.



