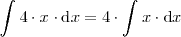Patrocinado por PHPDocX

Síguenos en Twitter

Buscar en WikilleratO
   

Propiedades de las integrales indefinidas

De Wikillerato

(Diferencias entre revisiones)
Revisión actual (16:37 11 jul 2013) (editar) (deshacer)
 
(29 ediciones intermedias no se muestran.)
Línea 1: Línea 1:
 +
<br/>
-
Por la definición, case derivada de la función integral indefinida es igual a la
+
==Propiedad 1==
-
función integrando:
+
<br/>
<br/>
Línea 7: Línea 7:
<center>
<center>
<math>
<math>
-
\left[
+
\int \mathrm{f}^\prime \left( \, x \, \right) \cdot \mathrm{d}x = \mathrm{f} \left( \,
-
\, \int \mathrm{f} \left( \, x \, \right) \mathrm{d}x \,
+
x \, \right) + C
-
\right]
+
-
^\prime \, = \, \mathrm{f} \left( \, x \, \right)
+
</math>
</math>
</center>
</center>
Línea 16: Línea 14:
<br/>
<br/>
-
La integral de la suma de dos funciones es igual a la suma de las integrales de las
+
==Propiedad 2==
-
funciones:
+
 
 +
<br/>
 +
 
 +
La integral de la suma de dos funciones es igual a la suma de las integrales de dichas funciones:
<br/>
<br/>
Línea 26: Línea 27:
\left(
\left(
\, \mathrm{f} \left( \, x \, \right) \, + \,
\, \mathrm{f} \left( \, x \, \right) \, + \,
-
\mathrm{g} \left( \, x \, \right) \cdot \mathrm{d}x
+
\mathrm{g} \left( \, x \, \right)
\right)
\right)
 +
\cdot \mathrm{d}x
\, = \,
\, = \,
\int \mathrm{f} \left( \, x \, \right) \cdot \mathrm{d}x \, + \,
\int \mathrm{f} \left( \, x \, \right) \cdot \mathrm{d}x \, + \,
-
\int \mathrm{f} \left( \, x \, \right) \cdot \mathrm{d}x
+
\int \mathrm{g} \left( \, x \, \right) \cdot \mathrm{d}x
</math>
</math>
-
</center>
+
</center>
<br/>
<br/>
-
La integral indefinida del producto de un n\'umero real &nbsp;
+
===Ejemplo===
 +
 
 +
<br/>
 +
 
 +
<center>
<math>
<math>
-
k
+
\int \left( \, x^2 + x \, \right) \cdot \mathrm{d}x =
 +
\int x^2 \cdot \mathrm{d}x +
 +
\int x \cdot \mathrm{d}x
</math>
</math>
-
&nbsp; por una función es igual al producto de &nbsp;
+
</center>
-
<math>
+
 
-
k
+
<br/>
-
</math>
+
 
-
&nbsp; por la integral indefinida de la función:
+
==Propiedad 3==
 +
 
 +
<br/>
 +
 
 +
La integral indefinida del producto de un número real &nbsp; <math> k </math> &nbsp; por una función <math> \mathrmf{f} </math> es igual al producto de &nbsp; <math> k </math> &nbsp; por la integral indefinida de <math> \mathrmf{f} </math>:
<br/>
<br/>
Línea 52: Línea 64:
\int k \cdot \mathrm{f} \left( \, x \, \right) \cdot \mathrm{d}x \, = \,
\int k \cdot \mathrm{f} \left( \, x \, \right) \cdot \mathrm{d}x \, = \,
k \cdot \int \mathrm{f} \left( \, x \, \right) \cdot \mathrm{d}x
k \cdot \int \mathrm{f} \left( \, x \, \right) \cdot \mathrm{d}x
 +
</math>
 +
</center>
 +
 +
 +
<br/>
 +
 +
===Ejemplo===
 +
 +
<br/>
 +
 +
<center>
 +
<math>
 +
\int 4 \cdot x \cdot \mathrm{d}x =
 +
4 \cdot \int x \cdot \mathrm{d}x
</math>
</math>
</center>
</center>
Línea 57: Línea 83:
<br/>
<br/>
-
Para demostrar las ultimas dos igualdades basta con derivar los dos terminos en
+
[[Category: Matemáticas]]
-
ambas igualdades.
+

Revisión actual


Tabla de contenidos

Propiedad 1


\int \mathrm{f}^\prime \left( \, x \, \right) \cdot \mathrm{d}x = \mathrm{f} \left( \, </p> <pre> x \, \right) + C </pre> <p>


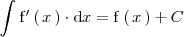
Propiedad 2


La integral de la suma de dos funciones es igual a la suma de las integrales de dichas funciones:


\int \left( </p> <pre> \, \mathrm{f} \left( \, x \, \right) \, + \, \mathrm{g} \left( \, x \, \right) </pre> <p>\right) \cdot \mathrm{d}x \, = \, \int \mathrm{f} \left( \, x \, \right) \cdot \mathrm{d}x \, + \, \int \mathrm{g} \left( \, x \, \right) \cdot \mathrm{d}x


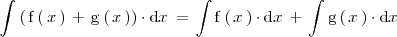
Ejemplo


\int \left( \, x^2 + x \, \right) \cdot \mathrm{d}x = \int x^2 \cdot \mathrm{d}x + \int x \cdot \mathrm{d}x


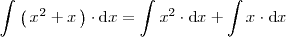
Propiedad 3


La integral indefinida  del producto de un número real     k     por una  función   \mathrmf{f}   es igual  al  producto de    k    por  la integral indefinida de   \mathrmf{f} :


\int k \cdot \mathrm{f} \left( \, x \, \right) \cdot \mathrm{d}x \, = \, </p> <pre>k \cdot \int \mathrm{f} \left( \, x \, \right) \cdot \mathrm{d}x </pre> <p>



Ejemplo


\int 4 \cdot x \cdot \mathrm{d}x = 4 \cdot \int x \cdot \mathrm{d}x


Obtenido de "http://wikillerato.org/Propiedades_de_las_integrales_indefinidas.html"
   
 
ASIGNATURAS
 MatemáticasFísicaQuímicaBiologíaDibujoHistoriaLengua y LiteraturaHistoria del ArteFilosofía
Creative Commons License
Los contenidos de Wikillerato están disponibles bajo una licencia de Creative Commons.
Pueden utilizarse y redistribuirse libremente siempre que se reconozca su procedencia.