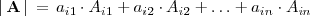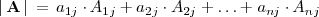Desarrollo de un determinante
De Wikillerato
m (Revertidas las ediciones realizadas por 200.118.90.180 (Talk); a la última edición de Laura.2mdc) |
|||
| (19 ediciones intermedias no se muestran.) | |||
| Línea 1: | Línea 1: | ||
En esta sección se explica un procedimiento que nos permite calcular determinantes de | En esta sección se explica un procedimiento que nos permite calcular determinantes de | ||
| - | cualquier orden, pero antes hemos de introducir los conceptos de '''''menor complementario''''' | + | cualquier orden, pero antes hemos de introducir los conceptos de '''''menor complementario''''' y '''''adjunto'''''. |
<br/> | <br/> | ||
| - | == | + | ==Menores complementarios y adjuntos== |
<br/> | <br/> | ||
| - | + | En una matriz cuadrada de orden | |
<math> | <math> | ||
| - | n, \, A = \left( \, a_{ij} \, \right), | + | n, \, \mathbf{A} = \left( \, a_{ij} \, \right), |
</math> | </math> | ||
se llama '''''menor complementario''''' del elemento | se llama '''''menor complementario''''' del elemento | ||
| Línea 35: | Línea 35: | ||
de la matriz | de la matriz | ||
<math> | <math> | ||
| - | A | + | \mathbf{A} |
</math> | </math> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Se llama '''''adjunto''''' del elemento | ||
| + | <math> | ||
| + | a_{ij} | ||
| + | </math>, | ||
| + | y lo representamos por | ||
| + | <math> | ||
| + | A_{ij}, | ||
| + | </math> | ||
| + | al producto | ||
| + | <math> | ||
| + | \left( \, -1 \, \right)^{i + j} \cdot \alpha_{ij} | ||
| + | </math>: | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | <center> | ||
| + | <math> | ||
| + | A_{ij} = \left( \, -1 \, \right)^{i + j} \cdot \alpha_{ij} | ||
| + | </math> | ||
| + | </center> | ||
<br/> | <br/> | ||
| Línea 50: | Línea 73: | ||
<center> | <center> | ||
<math> | <math> | ||
| - | A = | + | \mathbf{A} = |
\left( | \left( | ||
\begin{array}{ccc} | \begin{array}{ccc} | ||
| Línea 79: | Línea 102: | ||
8 & 9 | 8 & 9 | ||
\end{array} | \end{array} | ||
| - | \right| | + | \right| |
| - | & | + | & |
\qquad \alpha_{12} = | \qquad \alpha_{12} = | ||
\left| | \left| | ||
| Línea 88: | Línea 111: | ||
7 & 9 | 7 & 9 | ||
\end{array} | \end{array} | ||
| - | \right| | + | \right| |
& | & | ||
\qquad \alpha_{13} = | \qquad \alpha_{13} = | ||
| Línea 98: | Línea 121: | ||
\end{array} | \end{array} | ||
\right| | \right| | ||
| - | |||
\\ | \\ | ||
| - | & | + | & & |
\\ | \\ | ||
\alpha_{21} = | \alpha_{21} = | ||
| Línea 109: | Línea 131: | ||
8 & 9 | 8 & 9 | ||
\end{array} | \end{array} | ||
| - | \right| | + | \right| |
& | & | ||
\qquad \alpha_{22} = | \qquad \alpha_{22} = | ||
| Línea 118: | Línea 140: | ||
7 & 9 | 7 & 9 | ||
\end{array} | \end{array} | ||
| - | \right| | + | \right| |
& | & | ||
\qquad \alpha_{23} = | \qquad \alpha_{23} = | ||
| Línea 127: | Línea 149: | ||
7 & 8 | 7 & 8 | ||
\end{array} | \end{array} | ||
| - | \right| | + | \right| |
\end{array} | \end{array} | ||
</math> | </math> | ||
| Línea 144: | Línea 166: | ||
5 & 6 | 5 & 6 | ||
\end{array} | \end{array} | ||
| - | \right| | + | \right| |
& | & | ||
\qquad \alpha_{32} = | \qquad \alpha_{32} = | ||
| Línea 153: | Línea 175: | ||
4 & 6 | 4 & 6 | ||
\end{array} | \end{array} | ||
| - | \right| | + | \right| |
& | & | ||
\qquad \alpha_{33} = | \qquad \alpha_{33} = | ||
| Línea 162: | Línea 184: | ||
4 & 5 | 4 & 5 | ||
\end{array} | \end{array} | ||
| - | \right| | + | \right| |
\end{array} | \end{array} | ||
</math> | </math> | ||
| Línea 169: | Línea 191: | ||
<br/> | <br/> | ||
| - | + | y sus adjuntos son: | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
<br/> | <br/> | ||
| Línea 232: | Línea 198: | ||
<math> | <math> | ||
\begin{array}{ccccccccccc} | \begin{array}{ccccccccccc} | ||
| - | A_{11} & = & -3 | + | A_{11} & = & -3 & \qquad & A_{12} & = & ~~~6 & \qquad & A_{13} & = & -3 |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
\\ | \\ | ||
| - | ~~6 & -12 & ~~6 | + | A_{21} & = & ~~6 & \qquad & A_{22} & = & -12 & \qquad & A_{23} & = & ~~6 |
\\ | \\ | ||
| - | -3 & ~~~6 & -3 | + | A_{31} & = & -3 & \qquad & A_{32} & = & ~~~6 & \qquad & A_{33} & = & -3 |
| - | \end{array} | + | &\end{array} |
| - | + | ||
</math> | </math> | ||
</center> | </center> | ||
| Línea 270: | Línea 214: | ||
El determinante de una matriz cuadrada de orden <math> n </math> es igual a la suma de los productos de los elementos | El determinante de una matriz cuadrada de orden <math> n </math> es igual a la suma de los productos de los elementos | ||
| - | de una línea o columna cualquiera por sus adjuntos respectivos. | + | de una línea o columna cualquiera por sus adjuntos respectivos. Es decir: |
| + | |||
| + | <br/> | ||
| + | |||
<center> | <center> | ||
<math> | <math> | ||
| - | + | \left| \, \mathbf{A} \, \right| \, = \, a_{i1} \cdot A_{i1} + a_{i2} \cdot A_{i2} + \ldots + a_{in} \cdot A_{in} | |
</math> | </math> | ||
</center> | </center> | ||
<center> | <center> | ||
<math> | <math> | ||
| - | + | \left| \, \mathbf{A} \, \right| \, = \, a_{1j} \cdot A_{1j} + a_{2j} \cdot A_{2j} + \ldots + a_{nj} \cdot A_{nj} | |
</math> | </math> | ||
</center> | </center> | ||
| Línea 284: | Línea 231: | ||
<br/> | <br/> | ||
| - | |||
| - | |||
| - | |||
| - | |||
| - | |||
| - | |||
| - | |||
[[Category:Matemáticas]] | [[Category:Matemáticas]] | ||
Revisión actual
En esta sección se explica un procedimiento que nos permite calcular determinantes de cualquier orden, pero antes hemos de introducir los conceptos de menor complementario y adjunto.
Menores complementarios y adjuntos
En una matriz cuadrada de orden
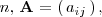 se llama menor complementario del elemento
se llama menor complementario del elemento
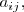 y lo representamos por
y lo representamos por
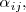 al determinante de la matriz cuadrada de orden
al determinante de la matriz cuadrada de orden
 que resulta de suprimir la fila
que resulta de suprimir la fila
 y la columna
y la columna
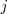 de la matriz
de la matriz
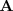
Se llama adjunto del elemento
 ,
y lo representamos por
,
y lo representamos por
 al producto
al producto
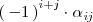 :
:
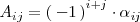
Ejemplo
Los menores complementarios de la matriz
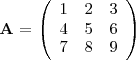
son
![\begin{array}{ccc}
\alpha_{11} =
\left|
</p>
<pre> \begin{array}[c]{cc}
5 & 6
\\
8 & 9
\end{array}
</pre>
<p>\right|
&
\qquad \alpha_{12} =
\left|
</p>
<pre> \begin{array}[c]{cc}
4 & 6
\\
7 & 9
\end{array}
</pre>
<p>\right|
&
\qquad \alpha_{13} =
\left|
</p>
<pre> \begin{array}[c]{cc}
4 & 5
\\
7 & 8
\end{array}
</pre>
<p>\right|
\\
& &
\\
\alpha_{21} =
\left|
</p>
<pre> \begin{array}[c]{cc}
2 & 3
\\
8 & 9
\end{array}
</pre>
<p>\right|
&
\qquad \alpha_{22} =
\left|
</p>
<pre> \begin{array}[c]{cc}
1 & 3
\\
7 & 9
\end{array}
</pre>
<p>\right|
&
\qquad \alpha_{23} =
\left|
</p>
<pre> \begin{array}[c]{cc}
1 & 2
\\
7 & 8
\end{array}
</pre>
<p>\right|
\end{array}
\begin{array}{ccc}
\alpha_{11} =
\left|
</p>
<pre> \begin{array}[c]{cc}
5 & 6
\\
8 & 9
\end{array}
</pre>
<p>\right|
&
\qquad \alpha_{12} =
\left|
</p>
<pre> \begin{array}[c]{cc}
4 & 6
\\
7 & 9
\end{array}
</pre>
<p>\right|
&
\qquad \alpha_{13} =
\left|
</p>
<pre> \begin{array}[c]{cc}
4 & 5
\\
7 & 8
\end{array}
</pre>
<p>\right|
\\
& &
\\
\alpha_{21} =
\left|
</p>
<pre> \begin{array}[c]{cc}
2 & 3
\\
8 & 9
\end{array}
</pre>
<p>\right|
&
\qquad \alpha_{22} =
\left|
</p>
<pre> \begin{array}[c]{cc}
1 & 3
\\
7 & 9
\end{array}
</pre>
<p>\right|
&
\qquad \alpha_{23} =
\left|
</p>
<pre> \begin{array}[c]{cc}
1 & 2
\\
7 & 8
\end{array}
</pre>
<p>\right|
\end{array}](/images/math/math-3783e29010f16c31576a73ebc6eb80c8.png)
![\begin{array}[c]{ccc}
\alpha_{31} =
\left|
</p>
<pre> \begin{array}[c]{cc}
2 & 3
\\
5 & 6
\end{array}
</pre>
<p>\right|
&
\qquad \alpha_{32} =
\left|
</p>
<pre> \begin{array}[c]{cc}
1 & 3
\\
4 & 6
\end{array}
</pre>
<p>\right|
&
\qquad \alpha_{33} =
\left|
</p>
<pre> \begin{array}[c]{cc}
1 & 2
\\
4 & 5
\end{array}
</pre>
<p>\right|
\end{array}
\begin{array}[c]{ccc}
\alpha_{31} =
\left|
</p>
<pre> \begin{array}[c]{cc}
2 & 3
\\
5 & 6
\end{array}
</pre>
<p>\right|
&
\qquad \alpha_{32} =
\left|
</p>
<pre> \begin{array}[c]{cc}
1 & 3
\\
4 & 6
\end{array}
</pre>
<p>\right|
&
\qquad \alpha_{33} =
\left|
</p>
<pre> \begin{array}[c]{cc}
1 & 2
\\
4 & 5
\end{array}
</pre>
<p>\right|
\end{array}](/images/math/math-03adb91ef28c3794b095ecb93b73fb77.png)
y sus adjuntos son:
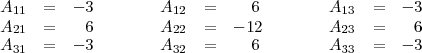
Desarrollo de un determinante
El determinante de una matriz cuadrada de orden  es igual a la suma de los productos de los elementos
de una línea o columna cualquiera por sus adjuntos respectivos. Es decir:
es igual a la suma de los productos de los elementos
de una línea o columna cualquiera por sus adjuntos respectivos. Es decir: