Matriz inversa
De Wikillerato
(→Ejemplo) |
m (Revertidas las ediciones realizadas por 201.134.78.78 (Talk); a la última edición de Laura.2mdc) |
||
| Línea 1: | Línea 1: | ||
__TOC__ | __TOC__ | ||
| + | ==Definición== | ||
| + | <br/> | ||
| + | |||
| + | La '''matriz inversa''' de una [[¿Qué es una matriz?|matriz]] cuadrada | ||
| + | <math> | ||
| + | A | ||
| + | </math> | ||
| + | de orden | ||
| + | <math> | ||
| + | n, | ||
| + | </math> | ||
| + | es la matriz, | ||
| + | <math> | ||
| + | A^{-1} | ||
| + | </math> | ||
| + | , de orden | ||
| + | <math> | ||
| + | n | ||
| + | </math> | ||
| + | que verifica: | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | <center> | ||
| + | <math> | ||
| + | A \cdot A^{-1} = A^{-1} \cdot A = I | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | donde | ||
| + | <math> | ||
| + | I | ||
| + | </math> | ||
| + | es la matriz identidad de orden | ||
| + | <math> | ||
| + | n | ||
| + | </math> | ||
| + | . | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Las matrices que tienen inversas se llaman regulares y las que no tienen inversa matrices | ||
| + | singulares. | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Las propiedades más importantes relativas a la matriz inversa: | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | 1. Si existe, | ||
| + | <math> | ||
| + | A^{-1} | ||
| + | </math> | ||
| + | es única. | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | 2. | ||
| + | <math> | ||
| + | \left( | ||
| + | A^{-1} | ||
| + | \right) | ||
| + | ^{-1} = A | ||
| + | </math> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | 3. | ||
| + | <math> | ||
| + | \left( | ||
| + | A \cdot B | ||
| + | \right) | ||
| + | ^{-1} = B^{-1} \cdot A^{-1} | ||
| + | </math> | ||
| + | |||
| + | <br/> | ||
==Cálculo de la matriz inversa== | ==Cálculo de la matriz inversa== | ||
| Línea 14: | Línea 93: | ||
<br/> | <br/> | ||
| + | ====Ejemplo==== | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | <center> | ||
| + | <math> | ||
| + | A = | ||
| + | \left( | ||
| + | \begin{array}[c]{cc} | ||
| + | 1 & 2 | ||
| + | \\ | ||
| + | 3 & 7 | ||
| + | \end{array} | ||
| + | \right) | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | hacemos | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | <center> | ||
| + | <math> | ||
| + | A^{-1} = | ||
| + | \left( | ||
| + | \begin{array}[c]{cc} | ||
| + | a & b</math> | ||
| + | </center> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | como | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | <center> | ||
| + | <math> | ||
| + | I = A \cdot A^{-1} \Rightarrow | ||
| + | \left( | ||
| + | \begin{array}[c]{cc} | ||
| + | 1 & 2 | ||
| + | \\ | ||
| + | 3 & 7 | ||
| + | \end{array} | ||
| + | \right) | ||
| + | \cdot | ||
| + | \left( | ||
| + | \begin{array}[c]{cc} | ||
| + | a & b | ||
| + | \\ | ||
| + | c & d | ||
| + | \end{array} | ||
| + | \right) | ||
| + | = | ||
| + | \left( | ||
| + | \begin{array}[c]{cc} | ||
| + | 1 & 0 | ||
| + | \\ | ||
| + | 0 & 1 | ||
| + | \end{array} | ||
| + | \right) | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Operando: | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | <center> | ||
| + | <math> | ||
| + | \left( | ||
| + | \begin{array}[c]{cc} | ||
| + | a + 2c & b + 2d | ||
| + | \\ | ||
| + | 3a + 7c & 3b + 7d | ||
| + | \end{array} | ||
| + | \right) | ||
| + | = | ||
| + | \left( | ||
| + | \begin{array}[c]{cc} | ||
| + | 1 & 0 | ||
| + | \\ | ||
| + | 0 & 1 | ||
| + | \end{array} | ||
| + | \right) | ||
| + | \Leftrightarrow | ||
| + | \left\{ | ||
| + | \begin{array}[c]{ccc} | ||
| + | a + 2c & = & 1 | ||
| + | \\ | ||
| + | 3a + 7c & = & 0 | ||
| + | \\ | ||
| + | b + 2d & = & 0 | ||
| + | \\ | ||
| + | 3b + 7d & = & 1 | ||
| + | \\ | ||
| + | \end{array} | ||
| + | \right. | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | <center> | ||
| + | <math> | ||
| + | \Rightarrow \left\{ | ||
| + | \begin{array}[c]{ccc} | ||
| + | a & = & 7 | ||
| + | \\ | ||
| + | b & = & -2 | ||
| + | \\ | ||
| + | c & = & -3 | ||
| + | \\ | ||
| + | d & = & 1 | ||
| + | \\ | ||
| + | \end{array} | ||
| + | \right. | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | ===Método de Gauss-Jordan=== | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | La inversa de una matriz regular | ||
| + | <math> | ||
| + | A | ||
| + | </math> | ||
| + | se calcular transformando la matriz | ||
| + | <math> | ||
| + | \left( | ||
| + | \, A \, \left| \, I \, \right. | ||
| + | \right) | ||
| + | </math> | ||
| + | mediante operaciones | ||
| + | elementales por filas en la matriz | ||
| + | <math> | ||
| + | \left( | ||
| + | \, I \, \left| \, A^{-1} \, \right. | ||
| + | \right) | ||
| + | </math> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | ====Operaciones elementales por filas en una matriz==== | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Las operaciones elementales por filas en una matriz son las siguientes: | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | 1. Intercambiar las filas | ||
| + | <math> | ||
| + | i | ||
| + | </math> | ||
| + | y | ||
| + | <math> | ||
| + | j, | ||
| + | </math> | ||
| + | que designaremos por | ||
| + | <math> | ||
| + | F_i \longrightarrow F_j | ||
| + | </math> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | 2. Multiplicar la fila | ||
| + | <math> | ||
| + | i | ||
| + | </math> | ||
| + | por el numero | ||
| + | <math> | ||
| + | k \neq 0 | ||
| + | </math> | ||
| + | y sustituirla por el resultado; lo designamos por | ||
| + | <math> | ||
| + | F_i \to k \cdot F_i | ||
| + | </math> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | 3. Sumar la fila | ||
| + | <math> | ||
| + | i | ||
| + | </math> | ||
| + | con la fila | ||
| + | <math> | ||
| + | j | ||
| + | </math> | ||
| + | y sustituirla por el resultado; lo designamos por | ||
| + | <math> | ||
| + | F_i \to \ F_i + F_j | ||
| + | </math> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | 4. Sumar las filas | ||
| + | <math> | ||
| + | i | ||
| + | </math> | ||
| + | y | ||
| + | <math> | ||
| + | j, | ||
| + | </math> | ||
| + | , multiplicadas por sendos números, y llevar el resultado a la fila | ||
| + | <math> | ||
| + | i | ||
| + | </math> | ||
| + | o | ||
| + | <math> | ||
| + | j | ||
| + | </math> | ||
| + | . Lo designamos por | ||
| + | <math> | ||
| + | F_i | ||
| + | </math> | ||
| + | o | ||
| + | <math> | ||
| + | F_j \to k \cdot F_i + t \cdot F_j | ||
| + | </math> | ||
Revisión de 07:35 27 may 2009
Tabla de contenidos |
Definición
La matriz inversa de una matriz cuadrada
 de orden
de orden
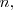 es la matriz,
es la matriz,
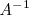 , de orden
, de orden
 que verifica:
que verifica:
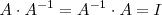
donde
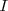 es la matriz identidad de orden
es la matriz identidad de orden
 .
.
Las matrices que tienen inversas se llaman regulares y las que no tienen inversa matrices singulares.
Las propiedades más importantes relativas a la matriz inversa:
1. Si existe,
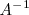 es única.
es única.
2.
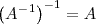
3.
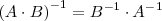
Cálculo de la matriz inversa
Para calcular la matriz inversa de una matriz regular podemos utilizar dos procedimientos:
Mediante la definicion
Ejemplo
![A =
\left(
</p>
<pre> \begin{array}[c]{cc}
1 & 2
\\
3 & 7
\end{array}
</pre>
<p>\right)
A =
\left(
</p>
<pre> \begin{array}[c]{cc}
1 & 2
\\
3 & 7
\end{array}
</pre>
<p>\right)](/images/math/math-1fe0b90602bd956d6622d417fadb9a6d.png)
hacemos
[Unparseable or potentially dangerous latex formula. Error 3 ]
como
![I = A \cdot A^{-1} \Rightarrow
\left(
</p>
<pre> \begin{array}[c]{cc}
1 & 2
\\
3 & 7
\end{array}
</pre>
<p>\right)
\cdot
\left(
</p>
<pre> \begin{array}[c]{cc}
a & b
\\
c & d
\end{array}
</pre>
<p>\right)
=
\left(
</p>
<pre> \begin{array}[c]{cc}
1 & 0
\\
0 & 1
\end{array}
</pre>
<p>\right)
I = A \cdot A^{-1} \Rightarrow
\left(
</p>
<pre> \begin{array}[c]{cc}
1 & 2
\\
3 & 7
\end{array}
</pre>
<p>\right)
\cdot
\left(
</p>
<pre> \begin{array}[c]{cc}
a & b
\\
c & d
\end{array}
</pre>
<p>\right)
=
\left(
</p>
<pre> \begin{array}[c]{cc}
1 & 0
\\
0 & 1
\end{array}
</pre>
<p>\right)](/images/math/math-83c4208e822a9057faf4bc22eb75b114.png)
Operando:
![\left(
</p>
<pre> \begin{array}[c]{cc}
a + 2c & b + 2d
\\
3a + 7c & 3b + 7d
\end{array}
</pre>
<p>\right)
=
\left(
</p>
<pre> \begin{array}[c]{cc}
1 & 0
\\
0 & 1
\end{array}
</pre>
<p>\right)
\Leftrightarrow
\left\{
</p>
<pre> \begin{array}[c]{ccc}
a + 2c & = & 1
\\
3a + 7c & = & 0
\\
b + 2d & = & 0
\\
3b + 7d & = & 1
\\
\end{array}
</pre>
<p>\right.
\left(
</p>
<pre> \begin{array}[c]{cc}
a + 2c & b + 2d
\\
3a + 7c & 3b + 7d
\end{array}
</pre>
<p>\right)
=
\left(
</p>
<pre> \begin{array}[c]{cc}
1 & 0
\\
0 & 1
\end{array}
</pre>
<p>\right)
\Leftrightarrow
\left\{
</p>
<pre> \begin{array}[c]{ccc}
a + 2c & = & 1
\\
3a + 7c & = & 0
\\
b + 2d & = & 0
\\
3b + 7d & = & 1
\\
\end{array}
</pre>
<p>\right.](/images/math/math-65ac35f7477a5f157f8f4b5954474b3d.png)
![\Rightarrow \left\{
</p>
<pre> \begin{array}[c]{ccc}
a & = & 7
\\
b & = & -2
\\
c & = & -3
\\
d & = & 1
\\
\end{array}
</pre>
<p>\right.
\Rightarrow \left\{
</p>
<pre> \begin{array}[c]{ccc}
a & = & 7
\\
b & = & -2
\\
c & = & -3
\\
d & = & 1
\\
\end{array}
</pre>
<p>\right.](/images/math/math-d80915bcc5f5cebafc112b3692daef76.png)
Método de Gauss-Jordan
La inversa de una matriz regular
 se calcular transformando la matriz
se calcular transformando la matriz
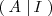 mediante operaciones
elementales por filas en la matriz
mediante operaciones
elementales por filas en la matriz
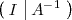
Operaciones elementales por filas en una matriz
Las operaciones elementales por filas en una matriz son las siguientes:
1. Intercambiar las filas
 y
y
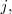 que designaremos por
que designaremos por

2. Multiplicar la fila
 por el numero
por el numero
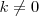 y sustituirla por el resultado; lo designamos por
[Unparseable or potentially dangerous latex formula. Error 3 ]
y sustituirla por el resultado; lo designamos por
[Unparseable or potentially dangerous latex formula. Error 3 ]
3. Sumar la fila
 con la fila
[Unparseable or potentially dangerous latex formula. Error 3 ]
y sustituirla por el resultado; lo designamos por
[Unparseable or potentially dangerous latex formula. Error 3 ]
con la fila
[Unparseable or potentially dangerous latex formula. Error 3 ]
y sustituirla por el resultado; lo designamos por
[Unparseable or potentially dangerous latex formula. Error 3 ]
4. Sumar las filas
 y
y
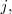 , multiplicadas por sendos números, y llevar el resultado a la fila
, multiplicadas por sendos números, y llevar el resultado a la fila
 o
o
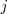 . Lo designamos por
. Lo designamos por
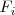 o
o

Véase también
Ejercicios resueltos
Producto e invertibilidad de matrices



