Cálculo de áreas y volúmenes
De Wikillerato
(Página nueva: axis) |
|||
| Línea 1: | Línea 1: | ||
| - | + | <br/> | |
| + | |||
| + | ==Cálculo de áreas mediante integrales== | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Supongamos que nos dan dos funciones | ||
| + | <math> | ||
| + | \mathrm{f} \left( \, x \, \right) | ||
| + | </math> | ||
| + | y | ||
| + | <math> | ||
| + | \mathrm{g} \left( \, x \, \right) | ||
| + | </math> | ||
| + | y nos preguntan calcular el área comprendida entre las graficas de ambas | ||
| + | funciones. | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | El area que nos piden coincide con el área comprendida entre la función | ||
| + | <math> | ||
| + | \mathrm{h} \left( \, x \, \right) := \mathrm{f} \left( \, x \, \right) - | ||
| + | \mathrm{g} \left( \, x \, \right) | ||
| + | </math> | ||
| + | y el eje X. | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Para calcular el área comprendida entre la función | ||
| + | <math> | ||
| + | \mathrm{h} | ||
| + | </math> | ||
| + | y el eje X, procedemos de la siguiente manera: | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | #1. En primer lugar resolvemos la ecuación: | ||
| + | |||
| + | <center> | ||
| + | <math> | ||
| + | \mathrm{h} \left( \, x \, \right) = 0 | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | para obtener | ||
| + | <math> | ||
| + | n | ||
| + | </math> | ||
| + | soluciones | ||
| + | <math> | ||
| + | x_1, \, x_2, \, \ldots, \, x_n | ||
| + | </math> | ||
| + | con | ||
| + | <center> | ||
| + | <math> | ||
| + | x_1 < x_2 < \ldots < x_n | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | #2 Buscamos una primitiva | ||
| + | <math> | ||
| + | \mathrm{H} \left( \, x \, \right) | ||
| + | </math> | ||
| + | de | ||
| + | <math> | ||
| + | \mathrm{h} \left( \, x \, \right) | ||
| + | </math>. | ||
| + | |||
| + | #3. Llegados a este punto ya podemos calcular el área que nos piden con la | ||
| + | fórmula: | ||
| + | <center> | ||
| + | <math> | ||
| + | \text{Area} = \left| \, \mathrm{H} \left( \, x_1 \, \right) - \mathrm{H} \left( | ||
| + | \, x_2 \, \right) \, \right| + | ||
| + | \left| \, \mathrm{H} \left( \, x_2 \, \right) - \mathrm{H} \left( | ||
| + | \, x_3 \, \right) \, \right| + \ldots + \left| \, \mathrm{H} \left( \, x_{n-1} \, \right) - \mathrm{H} \left( | ||
| + | \, x_n \, \right) \, \right| | ||
| + | </math> | ||
| + | </center> | ||
| + | donde | ||
| + | <center> | ||
| + | <math> | ||
| + | \left| \, \mathrm{H} \left( \, x_{i-1} \, \right) - \mathrm{H} \left( | ||
| + | \, x_i \, \right) \, \right| | ||
| + | </math> | ||
| + | </center> | ||
| + | es el area comprendida entre las rectas verticales de ecuaciones | ||
| + | <math> | ||
| + | x = x_{i-1} | ||
| + | </math>, | ||
| + | | ||
| + | <math> | ||
| + | x = x_i | ||
| + | </math>, | ||
| + | el eje X y la grafica de la función | ||
| + | <math> | ||
| + | \mathrm{h} | ||
| + | </math>. | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | ===Ejemplo=== | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Calculemos el área comprendida entre las graficas de | ||
| + | <math> | ||
| + | \mathrm{f} \left( \, x \, \right) = x^3 | ||
| + | </math> | ||
| + | y | ||
| + | <math> | ||
| + | \mathrm{g} \left( \, x \, \right) = x | ||
| + | </math>. | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | El area que nos piden coincide con el área comprendida entre la función | ||
| + | <math> | ||
| + | \mathrm{h} \left( \, x \, \right) := \mathrm{f} \left( \, x \, \right) - | ||
| + | \mathrm{g} \left( \, x \, \right) = x^3 - x | ||
| + | </math> | ||
| + | y el eje X. | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Para calcular el área comprendida entre la función | ||
| + | <math> | ||
| + | \mathrm{h} | ||
| + | </math> | ||
| + | y el eje X, procedemos de la siguiente manera: | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | #1. En primer lugar resolvemos la ecuación: | ||
| + | |||
| + | <center> | ||
| + | <math> | ||
| + | \mathrm{h} \left( \, x \, \right) = x^3 - x | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | para obtener 3 soluciones | ||
| + | soluciones | ||
| + | <math> | ||
| + | x_1 = -1, \, x_2 = 0, \, x_3 = 1 | ||
| + | </math>. | ||
| + | |||
| + | #2 Una primitiva | ||
| + | <math> | ||
| + | \mathrm{H} \left( \, x \, \right) | ||
| + | </math> | ||
| + | de | ||
| + | <math> | ||
| + | \mathrm{h} \left( \, x \, \right) | ||
| + | </math> | ||
| + | es | ||
| + | <center> | ||
| + | <math> | ||
| + | \mathrm{H} \left( \, x \, \right) = \frac{x^4}{4} - \frac{x^2}{2} | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | |||
| + | #3. Llegados a este punto ya podemos calcular el área que nos piden: | ||
| + | <center> | ||
| + | <math> | ||
| + | \text{Area} = \left| \, \mathrm{H} \left( \, x_1 \, \right) - \mathrm{H} \left( | ||
| + | \, x_2 \, \right) \, \right| + | ||
| + | \left| \, \mathrm{H} \left( \, x_2 \, \right) - \mathrm{H} \left( | ||
| + | \, x_3 \, \right) \, \right| = | ||
| + | \left| \, \mathrm{H} \left( \, -1 \, \right) - \mathrm{H} \left( | ||
| + | \, 0 \, \right) \, \right| + | ||
| + | \left| \, \mathrm{H} \left( \, 0 \, \right) - \mathrm{H} \left( | ||
| + | \, 1 \, \right) \, \right| = | ||
| + | \left| \, \frac{\left( \, -1 \, \right)^4}{4} - \frac{\left( \, -1 \, | ||
| + | \right)^3}{2} - \frac{\left( \, 0 \, \right)^4}{4} + \frac{\left( \, 0 \, | ||
| + | \right)^3}{2} \, \right| + | ||
| + | \left| \, \frac{\left( \, 0 \, \right)^4}{4} - \frac{\left( \, 0 \, | ||
| + | \right)^3}{2} - \frac{\left( \, 1 \, \right)^4}{4} + \frac{\left( \, 1 \, | ||
| + | \right)^3}{2} \, \right| | ||
| + | </math> | ||
| + | </center> | ||
Revisión de 10:47 25 dic 2010
Cálculo de áreas mediante integrales
Supongamos que nos dan dos funciones
 y
y
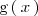 y nos preguntan calcular el área comprendida entre las graficas de ambas
funciones.
y nos preguntan calcular el área comprendida entre las graficas de ambas
funciones.
El area que nos piden coincide con el área comprendida entre la función
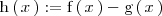 y el eje X.
y el eje X.
Para calcular el área comprendida entre la función
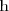 y el eje X, procedemos de la siguiente manera:
y el eje X, procedemos de la siguiente manera:
- 1. En primer lugar resolvemos la ecuación:

para obtener
 soluciones
soluciones
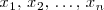 con
con
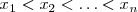
- 2 Buscamos una primitiva
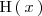 de
de
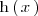 .
.
- 3. Llegados a este punto ya podemos calcular el área que nos piden con la
fórmula:

donde
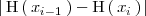
es el area comprendida entre las rectas verticales de ecuaciones
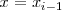 ,
,
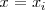 ,
el eje X y la grafica de la función
,
el eje X y la grafica de la función
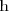 .
.
Ejemplo
Calculemos el área comprendida entre las graficas de
 y
y
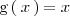 .
.
El area que nos piden coincide con el área comprendida entre la función
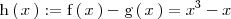 y el eje X.
y el eje X.
Para calcular el área comprendida entre la función
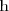 y el eje X, procedemos de la siguiente manera:
y el eje X, procedemos de la siguiente manera:
- 1. En primer lugar resolvemos la ecuación:
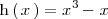
para obtener 3 soluciones
soluciones
 .
.
- 2 Una primitiva
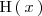 de
de
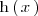
es
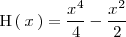
- 3. Llegados a este punto ya podemos calcular el área que nos piden:
[Unparseable or potentially dangerous latex formula. Error 1 ]



