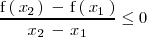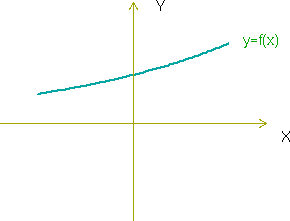Funciones crecientes y decrecientes
De Wikillerato
(→Función decreciente en un intervalo) |
|||
| (71 ediciones intermedias no se muestran.) | |||
| Línea 1: | Línea 1: | ||
| - | ==Función | + | ==Función estrictamente creciente en un intervalo== |
| - | + | <br/> | |
| - | + | Una función | |
| + | <math> | ||
| + | \mathrm{f} \left( \, x \, \right) | ||
| + | </math> | ||
| + | es '''''estrictamente creciente''''' en un intervalo | ||
| + | <math> | ||
| + | \left( | ||
| + | \, a, \, b \, | ||
| + | \right) | ||
| + | </math> | ||
| + | , si para dos valores cualesquiera del intervalo, | ||
| + | <math> | ||
| + | x_1 | ||
| + | </math> | ||
| + | y | ||
| + | <math> | ||
| + | x_2 | ||
| + | </math> | ||
| + | , se cumple que: | ||
| - | + | <br/> | |
| - | + | ||
| - | + | ||
| - | + | <center> | |
| + | <math> | ||
| + | \frac{\mathrm{f}\left( \, x_2 \, \right) \, - \, \mathrm{f}\left( \, x_1 \, \right)}{x_2 | ||
| + | \, - \, x_1} > 0 | ||
| + | </math> | ||
| + | </center> | ||
| - | + | <br/> | |
| - | + | | |
| - | + | ||
| - | + | ||
| - | + | <br/> | |
| - | + | <center> | |
| - | + | [[Imagen:funcion4.png]] | |
| - | + | </center> | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | <br/> | |
| - | + | Cuando en la gráfica de una función estrictamente creciente nos movemos hacia la derecha | |
| - | + | tambien nos movemos hacia arriba: | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | <br/> | |
| - | + | <center> | |
| - | se | + | <math> |
| + | x_2 > x_1 \Rightarrow | ||
| + | \mathrm{f} \left( \, x_2 \, \right) > \mathrm{f} \left( \, x_1 \, \right) | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Una función | ||
| + | <math> | ||
| + | f | ||
| + | </math> | ||
| + | es estrictamente creciente en el punto de abcisa | ||
| + | <math> | ||
| + | x \, = \, a | ||
| + | </math> | ||
| + | si existe algun número positivo | ||
| + | <math> | ||
| + | h | ||
| + | </math> | ||
| + | tal que | ||
| + | <math> | ||
| + | \mathrm{f} | ||
| + | |||
| + | </math> | ||
| + | es estrictamente creciente en el intervalo | ||
| + | <math> | ||
| + | \left( | ||
| + | \, x \, - \, h, \, x \, + \, h \, | ||
| + | \right) | ||
| + | </math>. | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | De esta esta definición se deduce que si | ||
| + | <math> | ||
| + | \mathrm{f} | ||
| + | </math> | ||
| + | es [[Definición de derivada|derivable]] en | ||
| + | <math> | ||
| + | x \, = \, a | ||
| + | </math> | ||
| + | y | ||
| + | <math> | ||
| + | f | ||
| + | </math> | ||
| + | es estrictamente creciente en el punto de abcisa | ||
| + | <math> | ||
| + | x \, = \, a | ||
| + | </math> | ||
| + | , entonces | ||
| + | <math> | ||
| + | \mathrm{f}^\prime \left( \, a \, \right) \ge 0 | ||
| + | </math>. | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | ==Función creciente en un intervalo== | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Una función | ||
| + | <math> | ||
| + | \mathrm{f} \left( \, x \, \right) | ||
| + | </math> | ||
| + | es '''''creciente''''' en un intervalo | ||
| + | <math> | ||
| + | \left( | ||
| + | \, a, \, b \, | ||
| + | \right) | ||
| + | </math> | ||
| + | , si para dos valores cualesquiera del intervalo, | ||
| + | <math> | ||
| + | x_1 | ||
| + | </math> | ||
| + | y | ||
| + | <math> | ||
| + | x_2 | ||
| + | </math> | ||
| + | , se cumple que: | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | <center> | ||
| + | <math> | ||
| + | \frac{\mathrm{f}\left( \, x_2 \, \right) \, - \, \mathrm{f}\left( \, x_1 \, \right)}{x_2 | ||
| + | \, - \, x_1} \ge 0 | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | ==Función estrictamente decreciente en un intervalo== | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Una función | ||
| + | <math> | ||
| + | \mathrm{f} \left( \, x \, \right) | ||
| + | </math> | ||
| + | es '''''estrictamente decreciente''''' en un intervalo | ||
| + | <math> | ||
| + | \left( | ||
| + | \, a, \, b \, | ||
| + | \right) | ||
| + | </math> | ||
| + | , si para dos valores cualesquiera del intervalo, | ||
| + | <math> | ||
| + | x_1 | ||
| + | </math> | ||
| + | y | ||
| + | <math> | ||
| + | x_2 | ||
| + | </math> | ||
| + | , se cumple que: | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | <center> | ||
| + | <math> | ||
| + | \frac{\mathrm{f}\left( \, x_2 \, \right) \, - \, \mathrm{f}\left( \, x_1 \, \right)}{x_2 | ||
| + | \, - \, x_1} < 0 | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | <center> | ||
| + | [[Imagen:funcion5.png]] | ||
| + | </center> | ||
| + | |||
| + | <br/> | ||
| + | |||
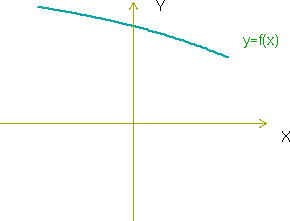
| + | Cuando en la gráfica de una función estrictamente decreciente nos movemos hacia la derecha | ||
| + | tambien nos movemos hacia abajo: | ||
| + | |||
| + | <center> | ||
| + | <math> | ||
| + | x_2 > x_1 \Rightarrow | ||
| + | \mathrm{f} \left( \, x_2 \, \right) < \mathrm{f} \left( \, x_1 \, \right) | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Una función | ||
| + | <math> | ||
| + | f | ||
| + | </math> | ||
| + | es estrictamente decreciente en el punto de abcisa | ||
| + | <math> | ||
| + | x \, = \, a | ||
| + | </math> | ||
| + | si existe algun número positivo | ||
| + | <math> | ||
| + | h | ||
| + | </math> | ||
| + | tal que | ||
| + | <math> | ||
| + | \mathrm{f} | ||
| + | |||
| + | </math> | ||
| + | es estrictamente decreciente en el intervalo | ||
| + | <math> | ||
| + | \left( | ||
| + | \, x \, - \, h, \, x \, + \, h \, | ||
| + | \right) | ||
| + | </math>. | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | De esta esta definición se deduce que si | ||
| + | <math> | ||
| + | \mathrm{f} | ||
| + | </math> | ||
| + | es [[Definición de derivada|derivable]] en | ||
| + | <math> | ||
| + | x \, = \, a | ||
| + | </math> | ||
| + | y | ||
| + | <math> | ||
| + | f | ||
| + | </math> | ||
| + | es estrictamente decreciente en el punto de abcisa | ||
| + | <math> | ||
| + | x \, = \, a | ||
| + | </math> | ||
| + | , entonces | ||
| + | <math> | ||
| + | \mathrm{f}^\prime \left( \, a \, \right) \le 0 | ||
| + | </math>. | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | ==Función decreciente en un intervalo== | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Una función | ||
| + | <math> | ||
| + | \mathrm{f} \left( \, x \, \right) | ||
| + | </math> | ||
| + | es '''''decreciente''''' en un intervalo | ||
| + | <math> | ||
| + | \left( | ||
| + | \, a, \, b \, | ||
| + | \right) | ||
| + | </math> | ||
| + | , si para dos valores cualesquiera del intervalo, | ||
| + | <math> | ||
| + | x_1 | ||
| + | </math> | ||
| + | y | ||
| + | <math> | ||
| + | x_2 | ||
| + | </math> | ||
| + | , se cumple que: | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | <center> | ||
| + | <math> | ||
| + | \frac{\mathrm{f}\left( \, x_2 \, \right) \, - \, \mathrm{f}\left( \, x_1 \, \right)}{x_2 | ||
| + | \, - \, x_1} \le 0 | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | |||
| + | == Véase también == | ||
| + | * [[Continuidad de una función en un punto]] | ||
| + | * [[Límite de una función]] | ||
| + | * [[Primitiva de una función]] | ||
| + | |||
| + | [[Category:Matemáticas]] | ||
Revisión actual
Tabla de contenidos |
Función estrictamente creciente en un intervalo
Una función
 es estrictamente creciente en un intervalo
es estrictamente creciente en un intervalo
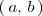 , si para dos valores cualesquiera del intervalo,
, si para dos valores cualesquiera del intervalo,
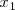 y
y
 , se cumple que:
, se cumple que:

Cuando en la gráfica de una función estrictamente creciente nos movemos hacia la derecha tambien nos movemos hacia arriba:
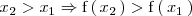
Una función
 es estrictamente creciente en el punto de abcisa
es estrictamente creciente en el punto de abcisa
 si existe algun número positivo
si existe algun número positivo
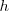 tal que
tal que
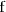 es estrictamente creciente en el intervalo
es estrictamente creciente en el intervalo
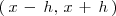 .
.
De esta esta definición se deduce que si
 es derivable en
es derivable en
 y
y
 es estrictamente creciente en el punto de abcisa
es estrictamente creciente en el punto de abcisa
 , entonces
, entonces
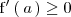 .
.
Función creciente en un intervalo
Una función
 es creciente en un intervalo
es creciente en un intervalo
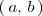 , si para dos valores cualesquiera del intervalo,
, si para dos valores cualesquiera del intervalo,
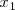 y
y
 , se cumple que:
, se cumple que:
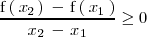
Función estrictamente decreciente en un intervalo
Una función
 es estrictamente decreciente en un intervalo
es estrictamente decreciente en un intervalo
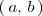 , si para dos valores cualesquiera del intervalo,
, si para dos valores cualesquiera del intervalo,
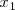 y
y
 , se cumple que:
, se cumple que:

Cuando en la gráfica de una función estrictamente decreciente nos movemos hacia la derecha tambien nos movemos hacia abajo:

Una función
 es estrictamente decreciente en el punto de abcisa
es estrictamente decreciente en el punto de abcisa
 si existe algun número positivo
si existe algun número positivo
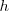 tal que
tal que
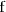 es estrictamente decreciente en el intervalo
es estrictamente decreciente en el intervalo
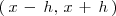 .
.
De esta esta definición se deduce que si
 es derivable en
es derivable en
 y
y
 es estrictamente decreciente en el punto de abcisa
es estrictamente decreciente en el punto de abcisa
 , entonces
, entonces
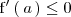 .
.
Función decreciente en un intervalo
Una función
 es decreciente en un intervalo
es decreciente en un intervalo
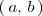 , si para dos valores cualesquiera del intervalo,
, si para dos valores cualesquiera del intervalo,
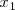 y
y
 , se cumple que:
, se cumple que: