Primitiva de una función
De Wikillerato
| (19 ediciones intermedias no se muestran.) | |||
| Línea 1: | Línea 1: | ||
| - | |||
==Definición== | ==Definición== | ||
| Línea 28: | Línea 27: | ||
\mathrm{f} \left( \, x \, \right) | \mathrm{f} \left( \, x \, \right) | ||
</math> | </math> | ||
| - | si la [[Definición de derivada|derivada]] de | + | en |
| + | <math> | ||
| + | I | ||
| + | </math> | ||
| + | si la [[Definición de derivada|derivada]] de | ||
<math> | <math> | ||
\mathrm{F} \left( \, x \, \right) | \mathrm{F} \left( \, x \, \right) | ||
| Línea 55: | Línea 58: | ||
| | ||
<math> | <math> | ||
| - | I \ | + | I \quad \Leftrightarrow \quad \left( \, \mathrm{F}^\prime \left( \, x \, \right) = |
| - | \mathrm{f} \left( \, x \, \right) | + | \mathrm{f} \left( \, x \, \right), \, \forall x \in I \, \right) |
</math> | </math> | ||
</center> | </center> | ||
| Línea 66: | Línea 69: | ||
<br/> | <br/> | ||
| - | ==Ejemplo== | + | ===Ejemplo=== |
<br/> | <br/> | ||
| Línea 131: | Línea 134: | ||
\mathrm{f} | \mathrm{f} | ||
</math> | </math> | ||
| - | , entonces existe un número | + | , entonces existe un número real |
<math> | <math> | ||
C | C | ||
</math> | </math> | ||
, tal que | , tal que | ||
| + | |||
| + | <br/> | ||
| + | |||
<center> | <center> | ||
<math> | <math> | ||
| Línea 145: | Línea 151: | ||
<br/> | <br/> | ||
| - | Reciprocamente, si a una primitiva de una | + | Reciprocamente, si a una primitiva de una función |
<math> | <math> | ||
\mathrm{f} | \mathrm{f} | ||
</math> | </math> | ||
| - | + | le añadimos una constante | |
| | ||
<math> | <math> | ||
| Línea 159: | Línea 165: | ||
</math> | </math> | ||
. | . | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | ===Ejemplo=== | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | | ||
| + | <math> | ||
| + | \mathrm{F} \left( \, x \, \right) \, = \, x^2 | ||
| + | </math> | ||
| + | y | ||
| + | <math> | ||
| + | \mathrm{G} \left( \, x \, \right) \, = \, x^2 \, + \, 7 | ||
| + | </math> | ||
| + | son dos funciones primitivas de | ||
| + | <math> | ||
| + | \mathrm{f}\left(\,x\,\right)\,=\,2\cdot x | ||
| + | </math>, | ||
| + | ya que | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | <center> | ||
| + | <math> | ||
| + | \mathrm{F}^\prime \left( \, x \, \right) \, = \, | ||
| + | \mathrm{G}^\prime \left( \, x \, \right) \, = \, | ||
| + | \mathrm{f} \left( \, x \, \right) | ||
| + | </math> | ||
| + | </center> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Observese que la diferencia | ||
| + | <math> | ||
| + | \mathrm{G} \left( \, x \, \right) \, - \, | ||
| + | \mathrm{F} \left( \, x \, \right) | ||
| + | </math> | ||
| + | es una constante ( = 7 ). | ||
| + | |||
| + | <br/> | ||
[[Category: Matemáticas]] | [[Category: Matemáticas]] | ||
Revisión actual
Tabla de contenidos |
Definición
Dadas dos funciones
 y
y
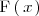 , definidas en un intervalo
, definidas en un intervalo
![</p>
<pre>I =
</pre>
<p>\left[
</p>
<pre> \, a, \, b \,
</pre>
<p>\right]
</p>
<pre>I =
</pre>
<p>\left[
</p>
<pre> \, a, \, b \,
</pre>
<p>\right]](/images/math/math-ec1b2022a1621b88d0396b296196a49e.png) , diremos que
, diremos que
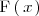 es una función primitiva de
es una función primitiva de
 en
en
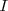 si la derivada de
si la derivada de
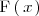 es la función
es la función
 en el intervalo
en el intervalo
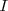 .
.
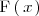 es primitiva de
es primitiva de
 en
en
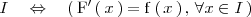
Calcular la primitiva de una función es el proceso inverso al de calcular su derivada.
Ejemplo
Consideremos la función
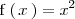 y denotemos por
y denotemos por
 la derivada de
la derivada de
 , es decir:
, es decir:

Entonces una primitiva de
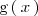 es
es
 .
.
¿Cuantas primitivas puede tener una función?
Una función cualquiera admite infinitas primitivas, de hecho
Dos funciones son primitivas de una misma función si y solo si se diferencian solo en una constante aditiva.
Es decir, si
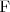 y
y
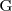 son primitivas de
son primitivas de
 , entonces existe un número real
, entonces existe un número real
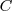 , tal que
, tal que
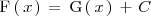
Reciprocamente, si a una primitiva de una función
 le añadimos una constante
le añadimos una constante
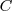 , entonces obtenemos otra primitiva de
, entonces obtenemos otra primitiva de
 .
.
Ejemplo
 y
y
 son dos funciones primitivas de
son dos funciones primitivas de
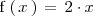 ,
ya que
,
ya que
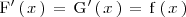
Observese que la diferencia
 es una constante ( = 7 ).
es una constante ( = 7 ).



