Extremos relativos
De Wikillerato
(→Ejercicios Resueltos) |
|||
| (14 ediciones intermedias no se muestran.) | |||
| Línea 1: | Línea 1: | ||
| - | == | + | ==Máximo relativo== |
<br/> | <br/> | ||
| Línea 5: | Línea 5: | ||
Una función | Una función | ||
<math> | <math> | ||
| - | \mathrm{f} | + | \mathrm{f} |
</math> | </math> | ||
| - | | + | alcanza un '''''máximo relativo''''' en el punto de abcisa |
<math> | <math> | ||
| - | + | x_0 | |
| - | + | ||
| - | + | ||
</math> | </math> | ||
| - | + | si existe un numero positivo | |
<math> | <math> | ||
| - | + | h | |
</math> | </math> | ||
| - | | + | de forma que |
<math> | <math> | ||
| - | + | \mathrm{f} \left( \, x_0 \, \right) > \mathrm{f} \left( \, x \, \right) | |
</math> | </math> | ||
| - | |||
| - | |||
| - | |||
| - | |||
| - | |||
| - | |||
| - | |||
| - | |||
| - | |||
| - | |||
| - | |||
| - | |||
| - | |||
| | ||
| - | + | para todos los puntos | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
<math> | <math> | ||
| - | + | x | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | x | + | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
</math> | </math> | ||
| - | | + | del intervalo |
<math> | <math> | ||
\left( | \left( | ||
| - | \, | + | \, x_0 \, - \, h, \, x_0 \, + \, h \, |
\right) | \right) | ||
</math>. | </math>. | ||
| Línea 84: | Línea 33: | ||
<br/> | <br/> | ||
| - | + | Si | |
<math> | <math> | ||
\mathrm{f} | \mathrm{f} | ||
| Línea 90: | Línea 39: | ||
es [[Definición de derivada|derivable]] en | es [[Definición de derivada|derivable]] en | ||
<math> | <math> | ||
| - | + | x_0 | |
</math> | </math> | ||
y | y | ||
<math> | <math> | ||
| - | f | + | \mathrm{f} |
</math> | </math> | ||
| - | | + | alcanza un máximo relativo en el punto de abcisa |
<math> | <math> | ||
| - | + | x_0 | |
</math> | </math> | ||
| - | + | entonces | |
<math> | <math> | ||
| - | \mathrm{f}^\prime \left( \, | + | \mathrm{f}^\prime \left( \, x_0 \, \right) \, = \, 0 |
</math>. | </math>. | ||
<br/> | <br/> | ||
| - | + | Si la función | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
<math> | <math> | ||
| - | \mathrm{f} | + | \mathrm{f} |
</math> | </math> | ||
| - | es | + | es [[Continuidad de una función|continua]], el que |
<math> | <math> | ||
| - | \ | + | \mathrm{f} |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
</math> | </math> | ||
| - | + | tenga un máximo relativo en un punto significa que la función es creciente a la | |
| + | izquierda y decreciente a la derecha de ese punto. | ||
<br/> | <br/> | ||
<center> | <center> | ||
| - | + | [[Imagen:maximo.png]] | |
| - | + | ||
| - | + | ||
| - | + | ||
</center> | </center> | ||
| - | |||
<br/> | <br/> | ||
| - | + | Si | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
<math> | <math> | ||
| - | \mathrm{f} \left( \, | + | \mathrm{f}^\prime \left( \, x_0 \, \right) \, = \, 0 |
</math> | </math> | ||
| - | | + | y |
<math> | <math> | ||
| - | \left( | + | \mathrm{f}^{\prime \prime} \left( \, x_0 \, \right) \, < \, 0 |
| - | + | ||
| - | \right) | + | |
</math> | </math> | ||
| - | + | entonces | |
<math> | <math> | ||
| - | + | \mathrm{f} | |
</math> | </math> | ||
| - | | + | tiene una máximo relativo en el punto de abcisa |
<math> | <math> | ||
| - | + | x \, = \, x_0 | |
| - | </math> | + | </math>. |
| - | + | ||
<br/> | <br/> | ||
| - | + | ==Mínimo relativo== | |
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
| - | + | ||
<br/> | <br/> | ||
| Línea 201: | Línea 99: | ||
Una función | Una función | ||
<math> | <math> | ||
| - | f | + | \mathrm{f} |
</math> | </math> | ||
| - | | + | alcanza un '''''mínimo relativo''''' en el punto de abcisa |
<math> | <math> | ||
| - | + | x_0 | |
</math> | </math> | ||
| - | si existe | + | si existe un numero positivo |
<math> | <math> | ||
h | h | ||
</math> | </math> | ||
| - | | + | de forma que |
<math> | <math> | ||
| - | \mathrm{f} | + | \mathrm{f} \left( \, x \, \right) > \mathrm{f} \left( \, x_0 \, \right) |
| - | + | </math> | |
| + | | ||
| + | para todos los puntos | ||
| + | <math> | ||
| + | x | ||
</math> | </math> | ||
| - | | + | del intervalo |
<math> | <math> | ||
\left( | \left( | ||
| - | \, | + | \, x_0 \, - \, h, \, x_0 \, + \, h \, |
\right) | \right) | ||
</math>. | </math>. | ||
| Línea 225: | Línea 127: | ||
<br/> | <br/> | ||
| - | + | Si | |
<math> | <math> | ||
\mathrm{f} | \mathrm{f} | ||
| Línea 231: | Línea 133: | ||
es [[Definición de derivada|derivable]] en | es [[Definición de derivada|derivable]] en | ||
<math> | <math> | ||
| - | + | x_0 | |
</math> | </math> | ||
y | y | ||
<math> | <math> | ||
| - | f | + | \mathrm{f} |
</math> | </math> | ||
| - | | + | alcanza un mínimo relativo en el punto de abcisa |
<math> | <math> | ||
| - | + | x_0 | |
</math> | </math> | ||
| - | + | entonces | |
<math> | <math> | ||
| - | \mathrm{f}^\prime \left( \, | + | \mathrm{f}^\prime \left( \, x_0 \, \right) \, = \, 0 |
</math>. | </math>. | ||
<br/> | <br/> | ||
| - | + | Si la función | |
| + | <math> | ||
| + | \mathrm{f} | ||
| + | </math> | ||
| + | es [[Continuidad de una función|continua]], el que | ||
| + | <math> | ||
| + | \mathrm{f} | ||
| + | </math> | ||
| + | tenga un mínimo relativo en un punto significa que la función es decreciente a la | ||
| + | izquierda y creciente a la derecha de ese punto. | ||
<br/> | <br/> | ||
| - | + | <center> | |
| + | [[Imagen:minimo.png]] | ||
| + | </center> | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | Si | ||
<math> | <math> | ||
| - | \mathrm{f} \left( \, | + | \mathrm{f}^{\prime} \left( \, x_0 \, \right) \, = \, 0 |
</math> | </math> | ||
| - | | + | y |
<math> | <math> | ||
| - | \left( | + | \mathrm{f}^{\prime \prime} \left( \, x_0 \, \right) \, > \, 0 |
| - | + | ||
| - | \right) | + | |
</math> | </math> | ||
| - | + | entonces | |
<math> | <math> | ||
| - | + | \mathrm{f} | |
</math> | </math> | ||
| - | | + | tiene una mínimo relativo en el punto de abcisa |
<math> | <math> | ||
| - | + | x \, = \, x_0 | |
| - | </math> | + | </math>. |
| - | + | ||
| - | <br/> | + | <br/> |
| + | |||
| + | ==Ejercicios Resueltos== | ||
| + | |||
| + | {{problemas}}* [http://www.selectividad.tv/S_M_2_1_20_S_asintotas_extremos_relativos_y_grafica_de_una_funcion.html Asíntotas, extremos relativos y gráfica de una función] | ||
| + | * [http://www.selectividad.tv/S_M_2_1_6_S_extremos_de_una_funcion_polinomica.html Extremos de una función polinómica] | ||
| - | |||
| - | |||
| - | |||
| - | |||
| - | |||
| - | |||
| - | |||
[[Category:Matemáticas]] | [[Category:Matemáticas]] | ||
Revisión actual
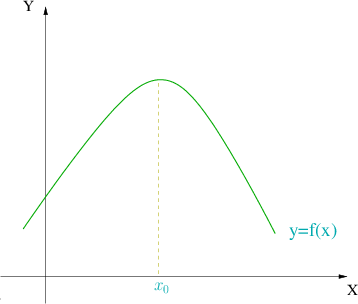
Máximo relativo
Una función
 alcanza un máximo relativo en el punto de abcisa
alcanza un máximo relativo en el punto de abcisa
 si existe un numero positivo
si existe un numero positivo
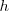 de forma que
de forma que
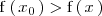 para todos los puntos
para todos los puntos
 del intervalo
del intervalo
 .
.
Si
 es derivable en
es derivable en
 y
y
 alcanza un máximo relativo en el punto de abcisa
alcanza un máximo relativo en el punto de abcisa
 entonces
entonces
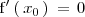 .
.
Si la función
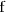 es continua, el que
es continua, el que
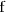 tenga un máximo relativo en un punto significa que la función es creciente a la
izquierda y decreciente a la derecha de ese punto.
tenga un máximo relativo en un punto significa que la función es creciente a la
izquierda y decreciente a la derecha de ese punto.
Si
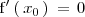 y
y
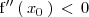 entonces
entonces
 tiene una máximo relativo en el punto de abcisa
tiene una máximo relativo en el punto de abcisa
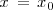 .
.
Mínimo relativo
Una función
 alcanza un mínimo relativo en el punto de abcisa
alcanza un mínimo relativo en el punto de abcisa
 si existe un numero positivo
si existe un numero positivo
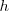 de forma que
de forma que
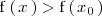 para todos los puntos
para todos los puntos
 del intervalo
del intervalo
 .
.
Si
 es derivable en
es derivable en
 y
y
 alcanza un mínimo relativo en el punto de abcisa
alcanza un mínimo relativo en el punto de abcisa
 entonces
entonces
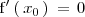 .
.
Si la función
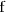 es continua, el que
es continua, el que
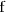 tenga un mínimo relativo en un punto significa que la función es decreciente a la
izquierda y creciente a la derecha de ese punto.
tenga un mínimo relativo en un punto significa que la función es decreciente a la
izquierda y creciente a la derecha de ese punto.
Si
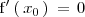 y
y
 entonces
entonces
 tiene una mínimo relativo en el punto de abcisa
tiene una mínimo relativo en el punto de abcisa
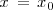 .
.
Ejercicios Resueltos
También te pueden interesar los siguientes ejercicios resueltos de selectividad