Procedimiento para factorizar un polinomio
De Wikillerato
(Diferencias entre revisiones)
(Página nueva: <br/> ==Procedimiento para factorizar un polinomio== <br/> # Sacamos <math> x </math> factor com\'un, si ello es posible. # Si el polinomio <math> \mathrm{P} \left( \, x \...) |
|||
| Línea 5: | Línea 5: | ||
<br/> | <br/> | ||
| - | + | 1. Sacamos <math> x </math> factor com\'un, si ello es posible. | |
| - | <math> | + | |
| - | x | + | |
| - | </math> | + | |
| - | factor com\'un, si ello es posible. | + | |
| - | + | <br/> | |
| + | |||
| + | 2. Si el polinomio | ||
<math> | <math> | ||
\mathrm{P} \left( \, x \, \right) | \mathrm{P} \left( \, x \, \right) | ||
| Línea 27: | Línea 25: | ||
</math> | </math> | ||
</center> | </center> | ||
| + | |||
Si esta ecuaci\'on no tiene solucion, el polinomio | Si esta ecuaci\'on no tiene solucion, el polinomio | ||
<math> | <math> | ||
| Línea 60: | Línea 59: | ||
\ldots + a_1 \cdot x + a_0 | \ldots + a_1 \cdot x + a_0 | ||
</math> | </math> | ||
| - | |||
| - | |||
| - | |||
| - | |||
| - | + | <br/> | |
| + | |||
| + | - es de grado mayor que dos | ||
| + | - sus coeficientes son enteros, y | ||
| + | - <math> \frac{a_0}{a_n} </math> es un entero | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | utilizamos regla de la Ruffini con los divisores de <math> | ||
\frac{a_0}{a_n} </math> y el polinomio | \frac{a_0}{a_n} </math> y el polinomio | ||
<math> | <math> | ||
| Línea 77: | Línea 80: | ||
\mathrm{P} \left( \, a \, \right) = 0 | \mathrm{P} \left( \, a \, \right) = 0 | ||
</math> | </math> | ||
| - | si y solo si | + | si y solo si br |
<math> | <math> | ||
x - a | x - a | ||
Revisión de 09:55 19 sep 2010
Procedimiento para factorizar un polinomio
1. Sacamos  factor com\'un, si ello es posible.
factor com\'un, si ello es posible.
2. Si el polinomio
 es de grado dos:
es de grado dos:
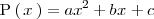
resolvemos la ecuaci\'on
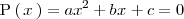
Si esta ecuaci\'on no tiene solucion, el polinomio
 es irreducible.
es irreducible.
Si las ecuaci\'on anterior tiene soluciones
 y
y
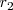 ,
entonces podemos factorizar
,
entonces podemos factorizar
 de la siguiente manera:
de la siguiente manera:
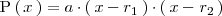
- Si el polinomio
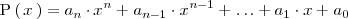
- es de grado mayor que dos
- sus coeficientes son enteros, y
- 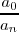 es un entero
es un entero
utilizamos regla de la Ruffini con los divisores de [Unparseable or potentially dangerous latex formula. Error 3 ] y el polinomio
 .
.
[Unparseable or potentially dangerous latex formula. Error 3 ]
si y solo si br
[Unparseable or potentially dangerous latex formula. Error 3 ]
es divisor de
 .
.



