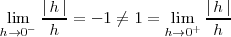Definición de derivada
De Wikillerato
(Diferencias entre revisiones)
| Línea 32: | Línea 32: | ||
\mathrm{f} | \mathrm{f} | ||
</math> | </math> | ||
| - | | + | NO es derivable en |
<math> | <math> | ||
x = a | x = a | ||
| Línea 114: | Línea 114: | ||
<br/> | <br/> | ||
| + | |||
| + | ==Ejemplo== | ||
| + | |||
| + | <br/> | ||
| + | |||
| + | La función | ||
| + | <math> | ||
| + | \mathrm{f} \left( \, x \, \right) = \left| \, x \, \right| | ||
| + | </math> | ||
| + | NO es derivable en | ||
| + | <math> | ||
| + | x = 0 | ||
| + | </math> | ||
| + | ya que no existe el limite | ||
| + | <center> | ||
| + | <math> | ||
| + | \lim_{h \to 0} \frac{\mathrm{f}\left( \, 0 \, + \, h \, \right) \, - \, | ||
| + | \mathrm{f} \left( \, 0 \, \right)}{h} | ||
| + | </math> | ||
| + | </center> | ||
| + | No existe por que | ||
| + | <center> | ||
| + | <math> | ||
| + | \lim_{h \to 0} \frac{\mathrm{f}\left( \, 0 \, + \, h \, \right) \, - \, | ||
| + | \mathrm{f} \left( \, 0 \, \right)}{h} = \lim_{h \to 0} \frac{\left| \, h \, \right|}{h} | ||
| + | </math> | ||
| + | </center> | ||
| + | y por que | ||
| + | <center> | ||
| + | <math> | ||
| + | \lim_{h \to 0^-} \frac{\left| \, h \, \right|}{h} = -1 \neq 1 = | ||
| + | \lim_{h \to 0^+} \frac{\left| \, h \, \right|}{h} | ||
| + | </math> | ||
| + | </center> | ||
[[Category:Matemáticas]] | [[Category:Matemáticas]] | ||
Revisión de 10:27 2 ene 2011
La derivada de la función
 en el punto
en el punto
 , si existe, es el valor del limite:
, si existe, es el valor del limite:
 .
.
Si este limite es un número real, la función
 es derivable en
es derivable en
 .
Si el límite anterior no es un número real o el límite no existe, la función
.
Si el límite anterior no es un número real o el límite no existe, la función
 NO es derivable en
NO es derivable en
 .
.
La derivada de la función
 en
en
 se denota por
se denota por
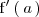 .
.
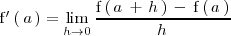 .
.
Ejemplo
Calculemos la derivada de
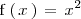 en
en
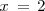 :
:
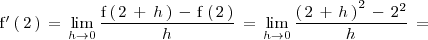
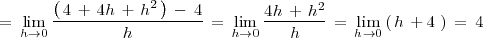
Ejemplo
La función
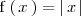 NO es derivable en
NO es derivable en
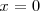 ya que no existe el limite
ya que no existe el limite

No existe por que
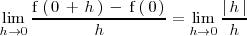
y por que