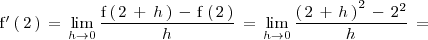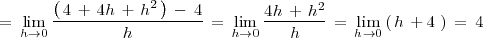Definición de derivada
De Wikillerato
(Diferencias entre revisiones)
| Línea 76: | Línea 76: | ||
\, = \, \lim_{h \to 0} \frac{\mathrm{f}\left( \, 2 \, + \, h \, \right) \, - \, | \, = \, \lim_{h \to 0} \frac{\mathrm{f}\left( \, 2 \, + \, h \, \right) \, - \, | ||
\mathrm{f} \left( \, 2 \, \right)}{h} \, = \, \lim_{h \to 0} \frac | \mathrm{f} \left( \, 2 \, \right)}{h} \, = \, \lim_{h \to 0} \frac | ||
| - | {\left( \, 2 \, + \, h \, \right)^2 \, - | + | {\left( \, 2 \, + \, h \, \right)^2 \, - \, 2^2}{h} \, = \, |
</math> | </math> | ||
| Línea 84: | Línea 84: | ||
\, = \, \lim_{h \to 0} | \, = \, \lim_{h \to 0} | ||
\frac {\left( \, 4 \, + \, 4h \, + \, h^2 \, \right) \, - \, 4}{h} \, = \, | \frac {\left( \, 4 \, + \, 4h \, + \, h^2 \, \right) \, - \, 4}{h} \, = \, | ||
| - | \lim_{h \to 0} \frac { | + | \lim_{h \to 0} \frac {4h \, + \, h^2}{h} \, = \, \lim_{h \to 0} |
| - | \lim_{h \to 0} | + | |
\left( | \left( | ||
\, h \, + 4 \, \, | \, h \, + 4 \, \, | ||
Revisión de 16:07 11 ene 2007
La derivada de la función
 en el punto
en el punto
 ,
,
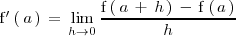 .
.
Si
 es un número real, la función
es un número real, la función
 es derivable en
es derivable en
 .
Si
.
Si
 no es un número real o el límite no existe, la función
no es un número real o el límite no existe, la función
 no es derivable en dicho punto.
no es derivable en dicho punto.
Ejemplo
Calculemos la derivada de
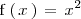 en
en
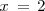 :
: