Indeterminaciones
De Wikillerato
Tabla de contenidos |
Introducción
Muchas de las funciones que se ven en bachillerato son continuas en toda la recta real o en casi todos los puntos de su dominio.
Este es el caso de los polinomios, las funciones exponenciales
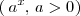 , el coseno, el seno, etc.
, el coseno, el seno, etc.
Si una función
 es continua en
es continua en
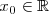 ,
el limite de
,
el limite de
 cuando
cuando
 tiende a
tiende a
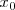 se puede calcular simplemente evaluando
se puede calcular simplemente evaluando
 en
en
 .
.
Ejemplo
Como
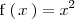 es una función continua en todo
es una función continua en todo
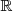 se tiene que
se tiene que
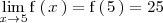
Indeterminación del tipo 0/0
En muchos casos, el limite se calcula utilizando las propiedades de los limites.
Por ejemplo, si existen los limites
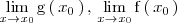
y
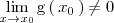
entonces se puede calcular el límite
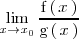
dividiendo
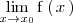 entre
entre
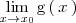 :
:
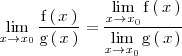
¿Pero que sucede cuando
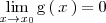 ?
?
Pueden darse dos casos:
- 1.
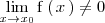 , o bien
, o bien
- 2.
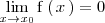 .
.
En este último caso, de exisir el limite
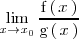
se ha de calcular de otra manera.
Procedimiento 1
Si  y
y
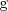 son polinomios, entonces se puede dividir ambos por
son polinomios, entonces se puede dividir ambos por
 ,
cuantas veces sea posible.
,
cuantas veces sea posible.
Ejemplo
Calculemos el limite
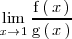
con
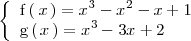
Ambos polinomios,
 y
y
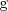 ,
se anulan en
,
se anulan en
 ,
por lo tanto ambos son divisibles por
,
por lo tanto ambos son divisibles por
 .
.
Si dividimos
 y
y
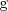 por
por
 una vez y luego otra, nos queda que
una vez y luego otra, nos queda que
[Unparseable or potentially dangerous latex formula. Error 3 ]
Todas esas divisiones se puede hacer por la regla de Ruffini.
Procedimiento 2
Independientemente de como sean
 y
y
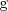 se puede utilizar la regla de L'Hôpital:
se puede utilizar la regla de L'Hôpital:
Si existe
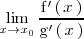
ya sea
 real, infinito o menos infinito, entonces
real, infinito o menos infinito, entonces
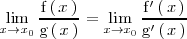 donde
donde
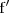 y
y
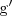 son las derivadas de
son las derivadas de
 y
y
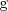 .
.
Ejemplo
Calculemos

Como la funcion seno y la funcion identidad
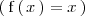 son funciones continuas, lo primero que hacemos es sustituir
son funciones continuas, lo primero que hacemos es sustituir
 por cero en
por cero en
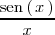
con lo que obtenemos la indeterminación
 .
.
Esto NO significa que el limite NO exista, de hecho si derivamos el numerador y
el denominador en
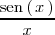 obtenemos
obtenemos
 que cuando
que cuando
 tiende a
tiende a
 tiende a 1.
tiende a 1.
Por lo tanto, por la regla de L'Hôpital
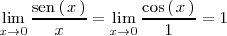
El ultimo límite se calcula teniendo en cuenta que la función coseno es continua




